Acids and Bases

Numerous definitions are being used by scientists when describing acids and bases. The simplest (and probably the most inaccurate) among them is that acids are generally sour, while bases are generally bitter.
For instance, citrus fruits are sour because they have citric acid, which, as its name suggests, is an acid. Vinegar is also sour because it is actually a dilute solution of acetic acid. Meanwhile, if you happened to accidentally swallow toothpaste while brushing your teeth, you may have noticed that it tastes bitter. If you like baking, at some point you’ve probably tasted baked goodies with a bitter aftertaste, which is probably due to excess baking soda added to the mix. This is because toothpaste and baking soda are both basic.
The problem with this method of classifying acids and bases is that it is inaccurate and can be life-threatening! Imagine tasting unknown substances just to determine if it is sour or bitter. In addition, there is no guarantee that a substance that tastes bitter will always be basic, while substances that taste sour will always be acidic. For example, brewed coffee tastes bitter, but when you measure its pH, most probably you will find it acidic.
Another problem with this definition is it does not offer any definitive and significant chemical distinction between acids and bases when compared to other groups of substances. Hence, scientists have devised a more elaborate definition to differentiate acids and bases.
There are numerous definitions so far, but in this chapter, we will focus our attention on the three most popular definitions in chemistry: Arrhenius, Bronsted-Lowry, and Lewis’s definitions of acids and bases.
Click below to go to the main reviewers:
Table of Contents
- The pH Scale
- Definition and Properties/Characteristics of Acids and Bases
- Strengths of Acids and Bases
- Acid/Base Strength and the Equilibrium Constant
- pH Calculations
- References
- Download Article in PDF Format
- Test Yourself!
The pH Scale
Before we proceed with the discussion, it is important that you know the pH scale. A typical pH scale has values that range from 1 to 14, wherein pH from 1 to 6.9 is considered acidic, while pH from 7.1 to 14 is considered basic. When the pH is exactly 7.0, it is said to be neutral.
pH values lower than 1 and higher than 14 are possible; however, we will not talk about that in this review material.
Definition and Properties/Characteristics of Acids and Bases
The most basic and probably the easiest definition to understand is the Arrhenius definition. The Arrhenius concept was proposed by Swedish chemist Svante Arrhenius in the 19th century. It states that acids produce hydrogen ions in an aqueous solution, while bases produce hydroxide ions.
Let’s take HCl and NaOH as examples. When dissolved in water, HCl dissociates completely according to the reaction:
HCl(aq) → H+(aq) + Cl—(aq)
As you can see, the dissociation of HCl in an aqueous solution yields hydrogen ions. Hence, HCl is an Arrhenius acid. However, you will seldom see the dissociation reaction of HCl written like this simply because H+ immediately reacts with H2O in the mixture, forming the hydronium ion, H3O+ according to the reaction
H+(aq) + H2O(l) → H3O+(aq)
Hence, in most references, you will see the dissociation reaction of HCl written as
HCl(aq) + H2O(l) → H3O+(aq) + Cl—(aq)
Other examples of Arrhenius acids include HNO3, H2SO4, HBr, HF, etc. Meanwhile, dissociation of NaOH in an aqueous solution happens according to the reaction
NaOH(s) → Na+(aq) + —OH(aq)
As seen, NaOH generates —OH in an aqueous solution, therefore it is considered an Arrhenius base. Other examples of Arrhenius bases are KOH, Mg(OH)2, Ca(OH)2, Al(OH)3, etc.
The limitation of Arrhenius’s definition is that it is applicable only to aqueous solutions. A broader definition was independently proposed by Johannes Bronsted and Thomas Lowry in 1923, now known as the Bronsted-Lowry definition. As defined, an acid is a proton donor, and a base is a proton acceptor.
Let us take NH3 as an example. You probably noticed that Arrhenius acids have ionizable hydrogen in the chemical formula, while Arrhenius bases have ionizable hydroxide in their chemical formula. If that’s the case, NH3 cannot be classified as Arrhenius acid or base because it does not have any ionizable H or OH in its formula. The Bronsted-Lowry definition, however, classifies NH3 as a base. In an aqueous solution, ammonia undergoes the equilibrium reaction shown below:
NH3(aq) + H2O(l) ⇌ NH4+(aq) + —OH(aq)
By closely inspecting the equilibrium reaction, we can see that NH3 accepts a proton from H2O to form NH4+ on the right side of the reaction. At the same time, H2O donates a proton to NH3, forming —OH. Therefore, based on definition, NH3 is a Bronsted-Lowry base (proton acceptor), while H2O is a Bronsted-Lowry acid (proton donor).
The tricky part is this: How about the reverse reaction? Which will serve as the acid and the base in the reverse reaction?
To avoid taking too much time reinspecting which species accepts and donates a proton, just remember that Bronsted-Lowry bases become an acid after it accepts a proton, while a Bronsted-Lowry acid becomes a base after it donates a proton. Therefore, since NH3 in our example is a base, its counterpart on the other side of the equation is an acid. Hence, NH4+ and NH3 are known as the conjugate acid-base pair.
Likewise, since water acts as the Bronsted-Lowry acid, its counterpart on the other side of the equation is a base. As a result, H2O and —OH is also conjugate acid-base pair. True enough, if the reverse reaction is considered, NH4+ donates a proton to —OH (hence, acting as an acid), while —OH accepts a proton from NH4+ (hence acting as a base) to form NH3 and H2O.
Lastly, the Lewis definition which was formulated by Gilbert N. Lewis in 1932 gives the most general definition of acids and bases. A Lewis base is a substance that can donate a pair of electrons, while a Lewis acid is a substance that can accept a pair of electrons. Let us apply this definition to our NH3 example, but to better illustrate, it is best to rewrite the reaction showing the Lewis structure of each species.
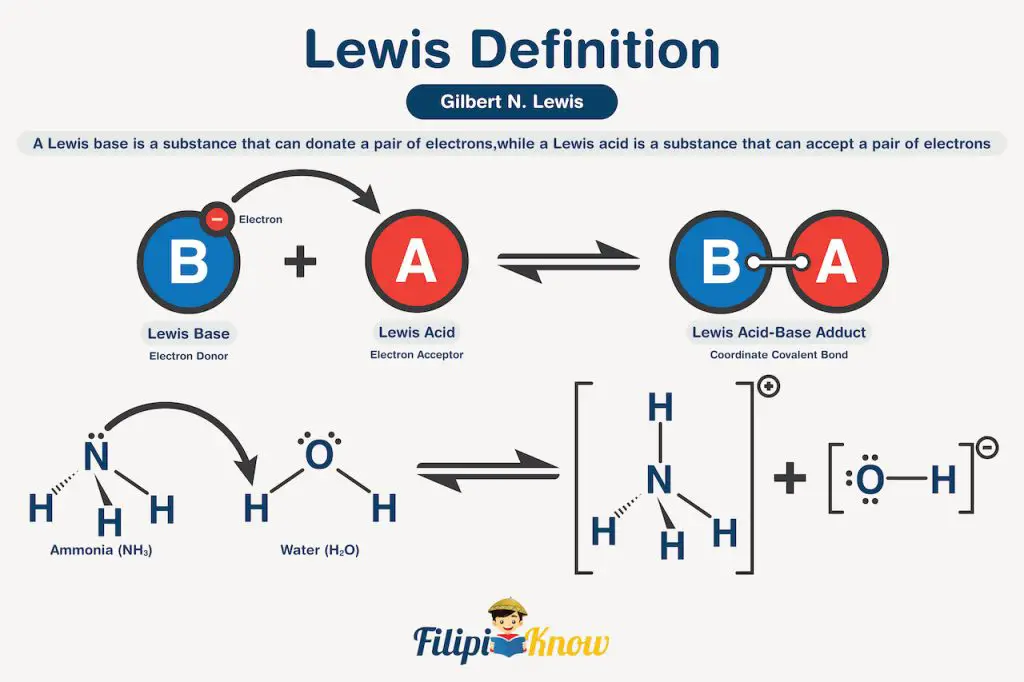
As you can see, the electron pair in the nitrogen atom of NH3 was donated to one of the hydrogen atoms of water, making NH3 a Lewis base. Meanwhile, the oxygen atom of water accepted the electron pair from one of the bonding electrons between oxygen and hydrogen, making H2O a Lewis acid.
Notice that the Bronsted-Lowry and Lewis definition consistently classifies water as acid and NH3 as a base. This is because Lewis’s definition is the most general definition among the three, while Arrhenius is the least. This means that all Arrhenius acids and bases are also Bronsted-Lowry acids and bases, and all Bronsted-Lowry acids and bases are also Lewis acids and bases. However, the reverse is not true.
Sample Problems:
Using the concept of Bronsted-Lowry, determine the conjugate acid-base pairs in the following chemical reactions.
- CH3COOH(aq) + H2O(l) ⇌ CH3COO—(aq) + H3O+(aq)
- CN—(aq) + H2O(l) ⇌ HCN(aq) + —OH(aq)
Solution:
The key to solving this problem is to determine which species on the left side of the equilibrium reaction donates and accepts a proton, then their counterpart on the other side will automatically be their conjugate. Let’s focus our attention first on the first problem.
CH3COOH(aq) + H2O(l) ⇌ CH3COO—(aq) + H3O+(aq)
As you can see, CH3COOH was converted to CH3COO—, a species that is one proton less relative to CH3COOH. This only means that the missing proton was donated somewhere. Hence, CH3COOH is a Bronsted-Lowry acid and its analog on the other side of the equation, the CH3COO—, will automatically be its conjugate base.
Now that we’ve identified the acid and its conjugate base, we can say even without inspection that H2O is acting as a base, while its conjugate acid is H3O+. To confirm this, note that H2O was converted to H3O+, a species that is one proton more than H2O itself. This means that H2O accepted a proton somewhere, hence, acting as a base.
The same approach can be applied to the reaction in number 2.
CN—(aq) + H2O(l) ⇌ HCN(aq) + —OH(aq)
In the forward reaction, CN— was converted to HCN, a species with one proton more than CN—. This means that CN— accepted a proton. Therefore, CN— acts as a base and HCN is its conjugate acid. Without further inspection, it can be concluded that H2O acts as an acid, and —OH is its conjugate base.
Strengths of Acids and Bases
Acids and bases can be classified based on their strength. In general, strong acids and bases are those species that dissociate completely in water, while weak acids and bases are those that only dissociate partially.
The hydroxides of Group I and Group II in the periodic table are considered strong bases. On the other hand, the following are considered strong acids: HCl, HBr, HI, HNO3, H2SO4 (first dissociation only), and HClO4. Aside from these, the rest are considered weak acids and bases.
When written as a reaction, you can immediately spot if a/an acid/base is weak or strong by looking at the arrows used. Dissociation of strong acids and bases uses a unidirectional arrow since the reaction goes to completion. Meanwhile, dissociation of weak acids and bases uses bidirectional harpoons to signify that the reaction is reversible and does not go to completion.
| Strong acid in water | HCl(aq) + H2O(l) → Cl—(aq) + H3O+(aq) |
| Weak acid in water | CH3COOH(aq) + H2O(l) ⇌ CH3COO—(aq) + H3O+(aq) |
| Strong base in water | NaOH(aq) → Na+(aq) + —OH(aq) |
| Weak base in water | NH3(aq) + H2O(l) ⇌ NH4+(aq) + —OH(aq) |
Take note that regardless of strength, an acid will always increase the [H3O+] in an aqueous solution, while a base will always increase the [—OH].
There are some species that can act both as an acid and base. Such species are called amphiprotic species or ampholytes. In fact, you’ve already seen one of these. In Sample Problem 1 in the previous section, water acts as a base, then in number 2, it acts as an acid!
However, don’t get confused. Water, in its pure state, is a neutral compound (pH = 7.0). Hence, it is neither an acid nor a base. But when a certain substance is added to it, it can act either as an acid or a base, depending on the nature of the added substance.
Another example is the bicarbonate ion, HCO3—.
| HCO3— acting as an acid | HCO3—(aq) + H2O(l) ⇌ CO32—(aq) + H3O+(aq) |
| HCO3— acting as a base | HCO3—(aq) + H2O(l) ⇌ H2CO3(aq) + —OH(aq) |
Acid/Base Strength and the Equilibrium Constant
The strength of weak acids and weak bases can be arranged by virtue of their equilibrium constants, Keq. Equilibrium constant expressions for dissociation of a weak acid and weak base are termed acid dissociation constant (designated as Ka) and base dissociation constant (designated as Kb), respectively.
Let’s consider the acetic acid, CH3COOH (Ka = 1.75 x 10—5).

As you can see from the Ka expression, it is directly proportional to the [H3O+]. This means that the higher the Ka value, the higher the proportion of the weak acid that is being ionized, and the stronger the weak acid.
However, there are instances that instead of Ka, you will be given pKa. pKa is simply equal to the negative logarithm of Ka. Since the relationship between exponential and logarithmic values is inverse, then we will reverse the relation. That is, the lower the pKa, the stronger the weak acid. This is also true for Kb and pKb.
Strong acids and bases also have Ka and Kb, respectively. However, its value is so large that it is usually not reported in Chemistry textbooks.
pH Calculations
One of the most distinguishing properties of acids and bases is that these compounds can alter the pH of the solution. A neutral solution has a pH of 7.0. Acidic solutions have a pH less than 7.0, while basic solutions have a pH greater than 7.0.
Since calculators are not allowed in your actual test, we will not solve for the exact pH of various acidic and basic solutions. However, you should know how to set up equations that will allow you to compute the pH of the solution since it is still possible to be asked in the test.
pH calculations of strong acids and bases are quite straightforward because the dissociation is complete. This means that if initially, you have 0.100 M of the reactant, this will all be converted to products as the reaction goes to completion.
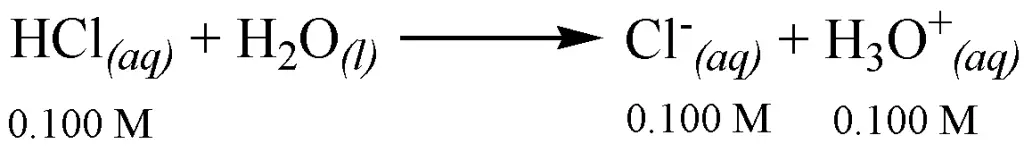
To compute the pH of acidic solutions, you simply take the negative logarithm of [H3O+]. Mathematically, that is:
pH = -log[H3O+]
Bases, on the other hand, release [—OH] in the solution. Hence, pH cannot be computed directly from the concentration of the base. Instead, pOH can be calculated by taking the negative logarithm of the [—OH].
pOH = -log[—OH]
Then at 25 ℃, pH + pOH = 14. Therefore, the pH of bases can be calculated by subtracting the calculated pOH by 14. If the temperature is not indicated in the problem, the standard practice is to assume that the temperature is 25 ℃.
Sample Problem:
Set up an equation that will allow us to determine the pH of 0.100 M Ca(OH)2.
Solution:
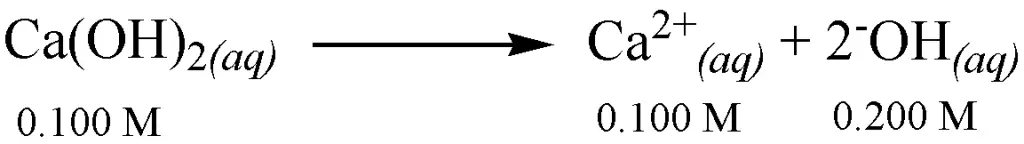
As you can see from the reaction above, a 0.100 M Ca(OH)2 solution liberates 0.200 M [—OH] since there are 2 moles of [—OH] per mole of Ca(OH)2. We can solve for the pOH of the solution using the expression
pOH = -log(0.200 M)
Then, to solve for pH:
pH = 14 – [-log(0.200 M)]
pH = 14 + log(0.200)
pH calculations become a little bit more complicated for weak acids and bases since these species do not dissociate completely. Take for example if we have 0.100 M NH3.
NH3(aq) + H2O(l) ⇌ NH4+(aq) + —OH(aq)
We know for a fact that NH3 is a weak Bronsted-Lowry base since it accepts a proton from water, thereby increasing the [—OH]. As you can see, the reaction is reversible. This implies that if initially, we have 0.100 M NH3, this doesn’t mean that we will also have 0.100 M —OH once the reaction reaches equilibrium. To address this kind of problem, we need to use our previous knowledge of equilibrium reactions and write equilibrium constant expressions. Furthermore, we can use the ICE table to help us solve equilibrium problems.
| Reaction | NH3(aq) | + | H2O(l) | ⇌ | NH4+(aq) | + | —OH(aq) |
| [Initial] | 0.100 | — | 0 | 0 | |||
| [Change] | -x | — | +x | +x | |||
| [Equilibrium] | 0.100 – x | — | x | x |
ICE table shows the initial concentration of all the reactants, the expected change in concentration, and the concentration of each species once the system reaches equilibrium.
Initially, we only have 0.100 M NH3 and no products are formed yet, so the initial concentration of the products is zero. As the reaction proceeds, the concentration of the reactant is expected to decline by a certain amount, hence we place -x since we do not know the exact amount at this point.
As the reactant is being used up, the products are also being formed, therefore, we place +x to both NH4+ and —OH because for every mole of NH3 that dissociates one mole of NH4+ and —OH is liberated. To obtain the equilibrium concentration, just add the initial concentration and the change in concentration.
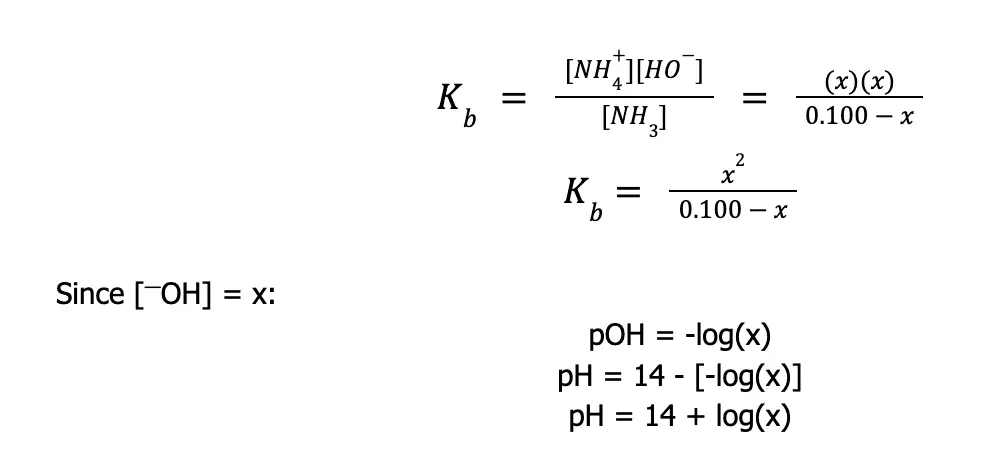
Normally, you can use the quadratic formula to solve for x, but since calculators are not allowed, we will limit our discussion to obtaining the expression/s necessary to solve the pH of solutions. Still, you can try solving this at home.
References
Chang, R. (2010). Chemistry (10th ed.). New York City, USA: McGraw-Hill Companies, Inc.
Kotz, J. (2022). Chemistry and Chemical Reactivity. Stamford, CT: Cengage Learning.
Silberberg, M. (2006). Chemistry: The molecular nature of matter and change (4th ed.). New York City, USA: McGraw-Hill Companies, Inc.
Zumdahl, S., Zumdahl, S., & DeCoste, D. (2010). Chemistry (8th ed.). Belmont, California: Brooks Cole.
Next topic: Thermochemistry
Previous topic: Chemical Equilibria
Return to the main article: The Ultimate Chemistry Reviewer
Download Article in PDF Format
Test Yourself!
1. Practice Questions [PDF Download]
2. Answer Key [PDF Download]
Written by John Bryan Rolloque
in College Entrance Exam, LET, NMAT, Reviewers, UPCAT
John Bryan Rolloque
John Bryan Rolloque graduated cum laude at the University of the Philippines Los Baños in 2018 under the B.S. Agricultural Chemistry program. He taught courses in general chemistry, analytical chemistry, and organic chemistry at UPLB’s Institute of Chemistry, and has been serving as the Region IV coordinator for the Regional and National Chemistry Olympiad. Landing 8th place in the 2019 licensure exam for agriculturists, he is now taking up his master’s degree in plant physiology, also in UPLB.
Copyright Notice
All materials contained on this site are protected by the Republic of the Philippines copyright law and may not be reproduced, distributed, transmitted, displayed, published, or broadcast without the prior written permission of filipiknow.net or in the case of third party materials, the owner of that content. You may not alter or remove any trademark, copyright, or other notice from copies of the content. Be warned that we have already reported and helped terminate several websites and YouTube channels for blatantly stealing our content. If you wish to use filipiknow.net content for commercial purposes, such as for content syndication, etc., please contact us at legal(at)filipiknow(dot)net
