An atom is the basic unit of an element that can enter into a chemical combination. Scientists are very interested in studying atoms and atomic structures simply because understanding atoms ultimately leads to understanding why certain elements behave or react in a certain manner.

In this section, we will study atomic theory as well as the different atomic properties, components, and models.

Click below to go to the main reviewers:

Ultimate UPCAT Reviewer

Ultimate NMAT Reviewer

Ultimate LET Reviewer

 

Table of Contents

• Atomic Theory of Matter
  • Hypothesis 1: All matter consists of indivisible particles called atoms
  • Hypothesis 2: All atoms of the same element are identical in terms of size, mass, and chemical properties. The atom of one element is different from the atom of another element
  • Hypothesis 3: Atoms of different elements may combine in fixed proportions to form a compound
  • Hypothesis 4: Chemical reactions involve reorganization of the atoms—changes in how they are bound together. The atoms themselves are not changed in a chemical reaction
• Atomic Models and Components of an Atom
• Properties of an Atom
• Quantum Mechanics
  • Quantum Numbers
    • 1. The Principal Quantum Number (n)
    • 2. Azimuthal Quantum Number (ℓ)
    • 3. Magnetic Quantum Number (mℓ)
    • 4. Spin Quantum Number (ms)
• Electron Configuration
  • The Aufbau Principle
• References
• Download Article in PDF Format
• Test Yourself!
  • 1. Practice Questions [PDF Download]
  • 2. Answer Key [PDF Download]

 

Atomic Theory of Matter

Can you imagine studying chemistry without any notion about atoms?

Well, people in the past experienced this kind of problem.

However, this doesn’t mean that past civilizations did not have an idea about the existence of atoms. The idea of an atom started as early as the 5th century B.C. when Democritus expressed his belief that matter is made up of smaller, indivisible particles he called ‘atomos,’ meaning indivisible.

The idea had been ignored for a long time until John Dalton formulated a precise definition of indivisible building blocks that we now call atoms in 1808. Dalton’s work laid the foundation for the modern era of chemistry. His hypotheses are enumerated below.

Hypothesis 1: All matter consists of indivisible particles called atoms

Dalton’s first hypothesis simply states that atoms are the smallest particles, and it is impossible to divide atoms even further. Of course, nowadays we know that this is not true, as many scientists have proven the existence of particles even smaller than the atom itself. We will discuss more of this in the next sections.

Hypothesis 2: All atoms of the same element are identical in terms of size, mass, and chemical properties. The atom of one element is different from the atom of another element

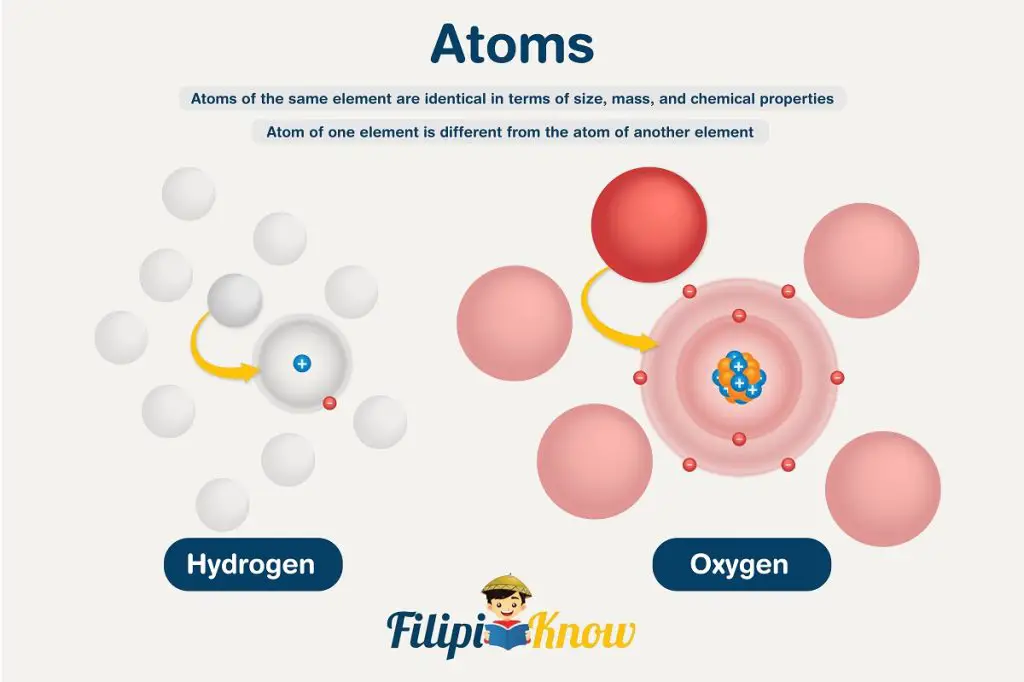
Simply put, this hypothesis suggests that hydrogen atoms are all the same. If you obtain hydrogen atoms from different parts of the world and in outer space, all the atoms will be the same in all respects. In the same way, oxygen atoms are all the same; however, oxygen atoms differ from hydrogen atoms. This hypothesis explains why hydrogen atoms behave similarly regardless of the source but differently with respect to oxygen atoms.

Hypothesis 3: Atoms of different elements may combine in fixed proportions to form a compound

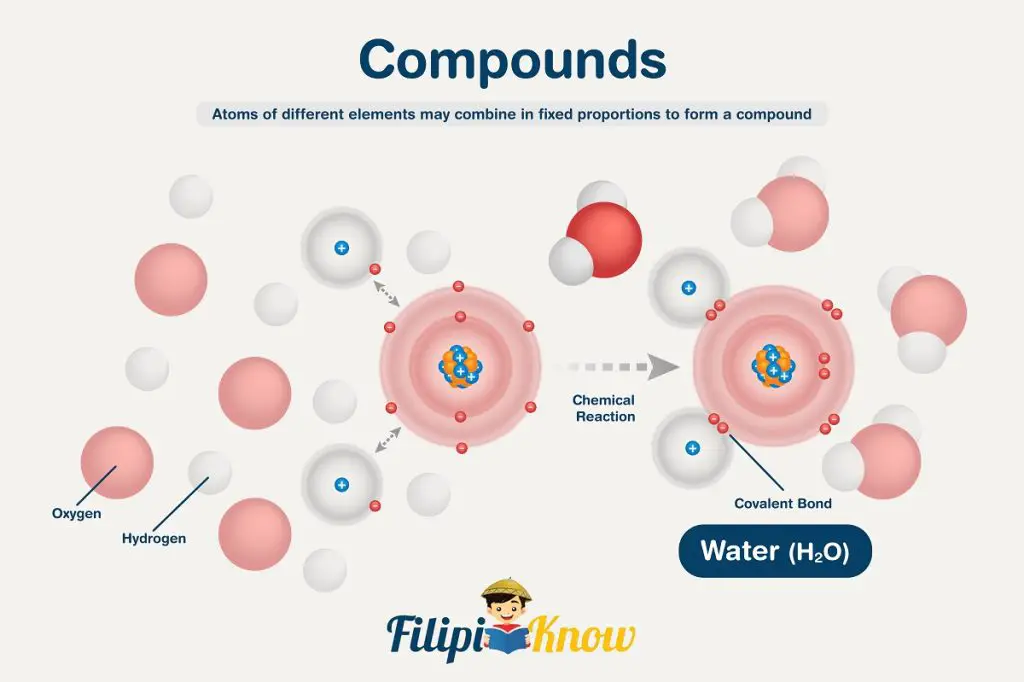
To visualize this theory, imagine a water molecule that is chemically written as H₂O. This chemical formula implies that ALL molecules of water are composed of two atoms of hydrogen and one atom of oxygen. Varying the proportion of at least one atom in the formula will give rise to a completely different compound.

Hypothesis 4: Chemical reactions involve reorganization of the atoms—changes in how they are bound together. The atoms themselves are not changed in a chemical reaction

This hypothesis is another way of stating the law of conservation of mass. In other words, atoms can neither be created nor destroyed, regardless of the type of chemical reaction it undergoes. Because atoms remain unchanged in a chemical reaction, the total mass of the reactants is equal to the total mass of the products after the reaction.

 

Atomic Models and Components of an Atom

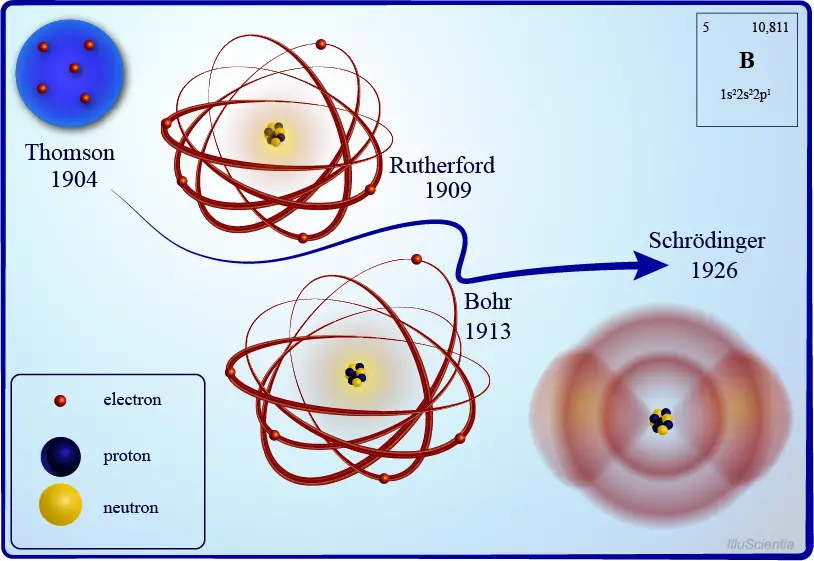
During his time, Dalton’s theory of the atom as the basic unit of an element that can participate in a chemical reaction was widely accepted. Advancements in modern technology, however, led to the discovery that atoms are made up of even smaller particles we now know as subatomic particles. These particles include protons, neutrons, and electrons.

Among the three subatomic particles, the electron was the very first to be discovered. In 1897, Sir Joseph John Thomson discovered the electron and through his cathode ray experiment, was able to determine that its charge-to-mass ratio is – 1.76 x 10⁸ C/g.

Since mass can never be negative, Sir Thomson’s findings suggest that electrons are negatively-charged. However, for an atom to be neutral (usually the case), there must be an equal number of positive and negative charges. This led Sir Thomson to propose that the atom is a uniform, positive sphere with electrons embedded in it like a raisin, giving rise to his plum pudding model of the atom.

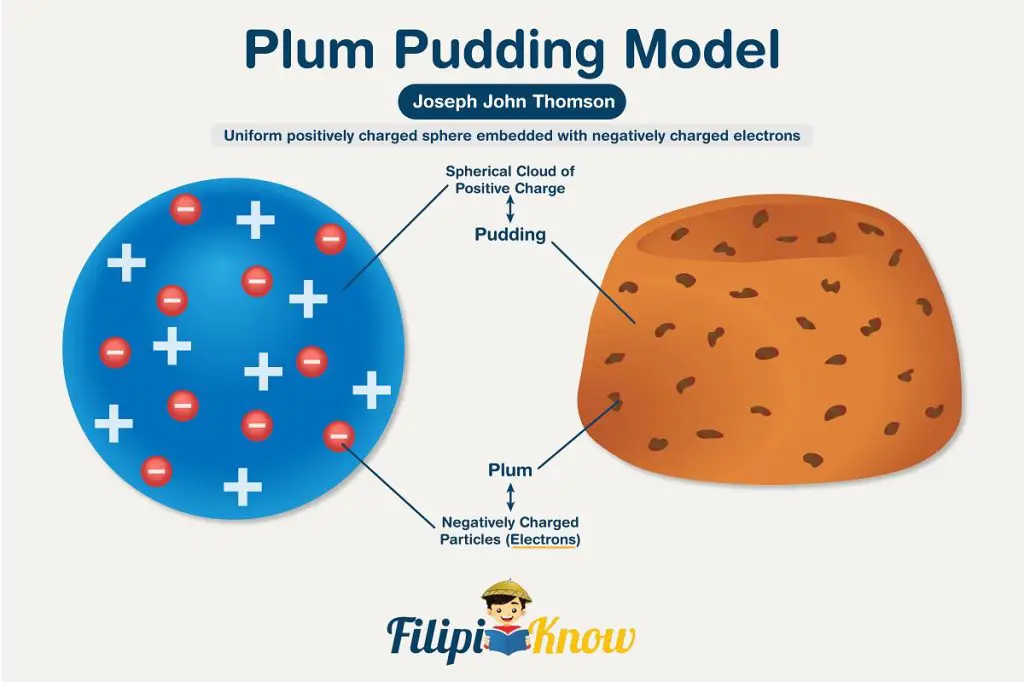
Years later, Robert Millikan’s oil drop experiment allowed him to calculate the charge of an electron, which he found to be – 1.6022 x 10^-19 C. Using his data and Thomson’s ratio, Millikan was able to derive the mass of a single electron, which is 9.10 x 10^-28 g!

The plum pudding model was the widely accepted model of the atom for a long time until Ernest Rutherford made the most surprising observation with his gold foil experiment.

In Rutherford and his colleagues’ series of experiments, they bombarded a very thin layer of gold with a positive ɑ particle. If Thomson’s model is correct, the diffused positive charge of the atom should have caused the ɑ particle to pass through the foil with very little deflection. Instead, Rutherford observed that the majority of the particles passed through the foil either completely undeflected or with very minimal deflection, while others deflected at a large angle and in extreme cases, bounced back to the direction where they came from! This observation paved the way for the new atomic model. 

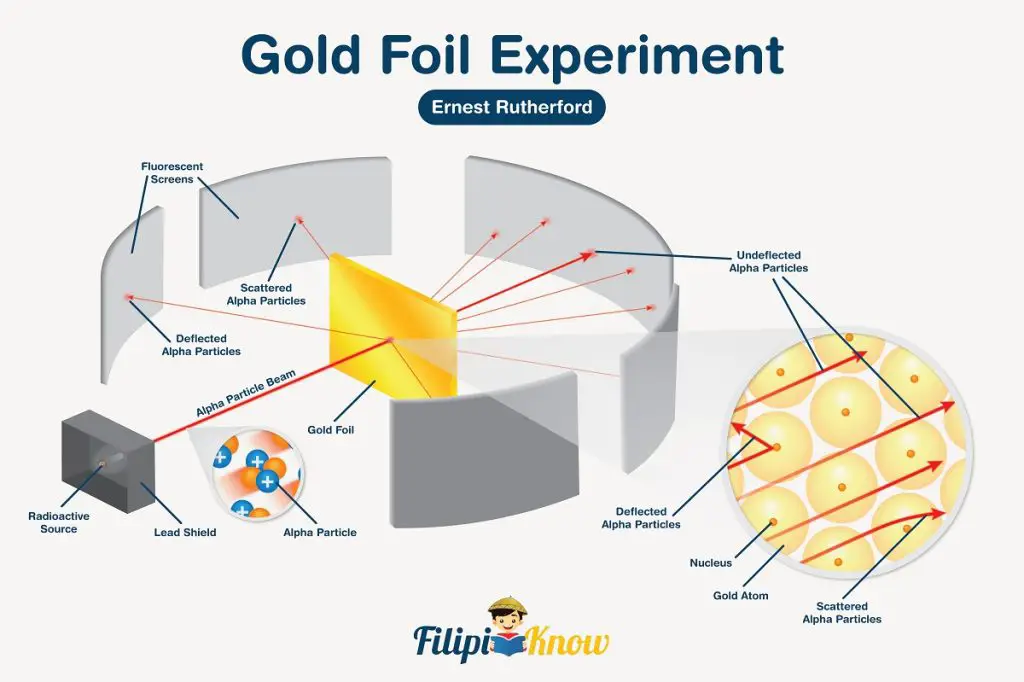
Rutherford inferred that atoms are mostly empty space. This is why most of the ɑ particles passed through the foil either undeflected or slightly deflected. As for the particles that either largely deflected or bounced back, Rutherford proposed that contrary to Thomson’s model, an atom has its positive charges concentrated in its core, which he called the nucleus.

As the positive ɑ particle approaches the positively-charged nucleus, it experiences repulsion due to similar charges, causing the particle to be deflected at such a large angle. Meanwhile, ɑ particles that directly hit the nucleus are deflected back towards the direction where they came from. Rutherford’s model gave rise to the atomic model that we know today!

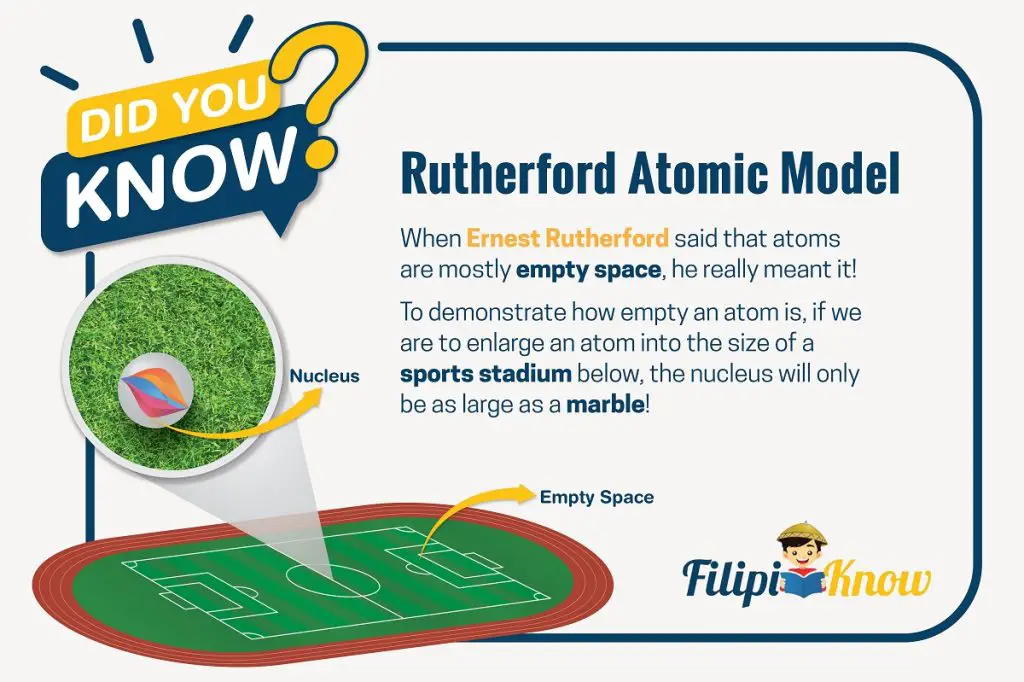
Rutherford’s proposed model, however, left an unsolved problem, specifically the mass ratio of hydrogen and helium. The atomic mass of hydrogen, an element with 1 proton and 1 electron, is 1.008 g/mole. Meanwhile, helium, an element with 2 protons and 2 electrons, has an atomic mass of 4.003. Therefore, the ratio of their atomic masses is about 1:4.

If protons and electrons are the only subatomic particles, their mass ratio should be 1:2 (the mass of electrons is usually omitted since it is 1840 times lighter than the proton!). In 1932, James Chadwick was able to account for this unexplained mass ratio due to his discovery of neutrons. Succeeding experiments proved that the third subatomic particle is electrically-neutral, hence the name neutron.

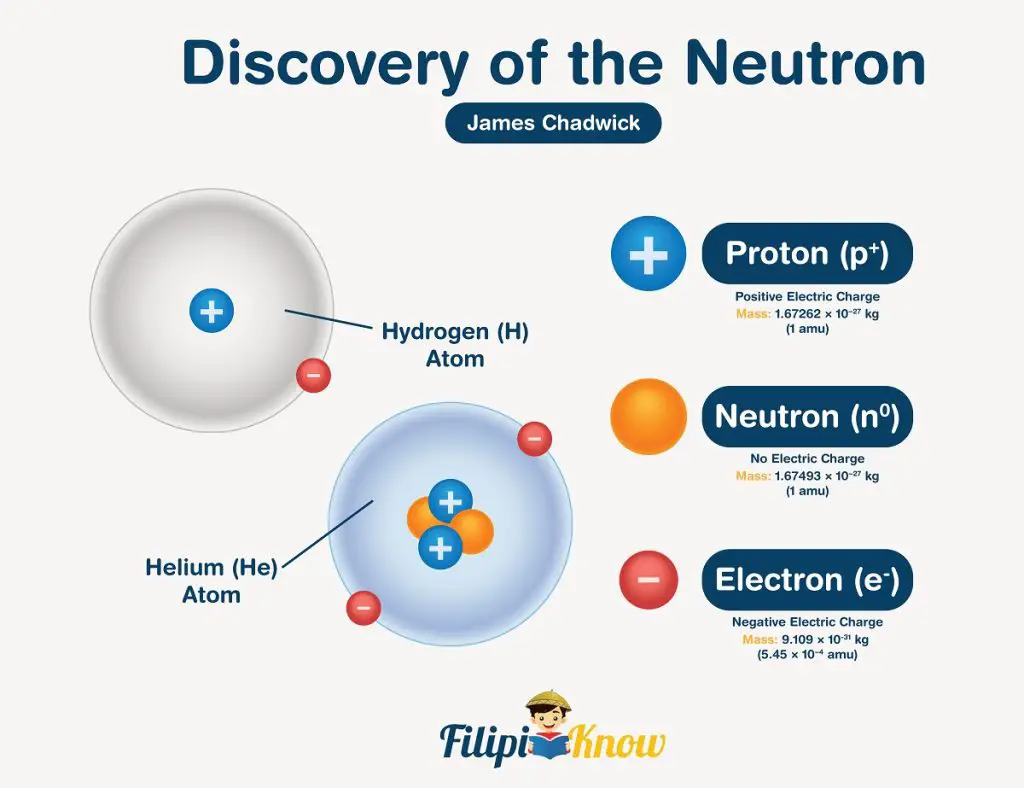
Particle	Mass (g)	Charge (Coulomb)	Charge unit
Proton	1.67262 x 10-24	1.6022 x 10-19	+1
Neutron	1.67493 x 10-24	0	0
Electron	9.10938 x 10-28	-1.6022 x 10-19	-1

 

Properties of an Atom

All the substances found on Earth are made up of atoms. If that’s the case, then how come a lot of substances vary significantly from one another?

The answer is because of the difference in the properties of the atom, which can be ultimately attributed to the number of protons and neutrons, as well as the number and distribution of electrons in the space around the nucleus.

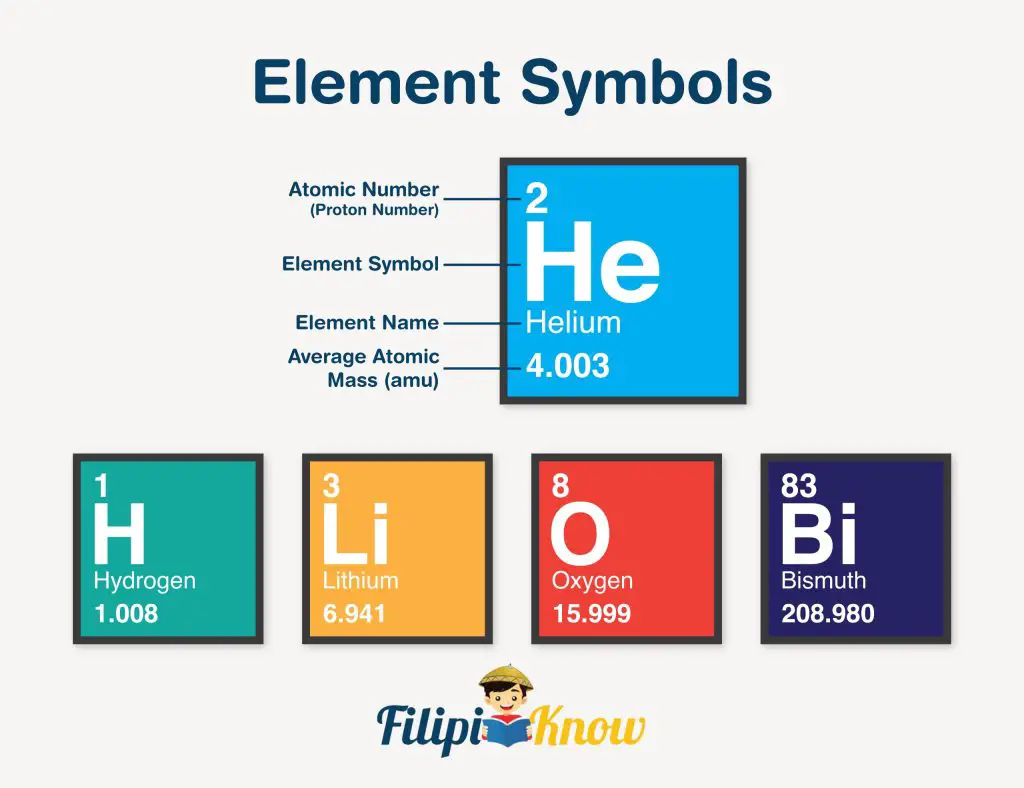
The first atomic property covered in this review is the atomic number (designated as Z). In the modern periodic table, an atomic number is usually written on the upper left side corner of each element block. This number represents the number of protons in the atom’s nucleus. It also gives us an idea about the reactivity of the atoms. For instance, all atoms with an atomic number of at least 84 are radioactive.

If the number of neutrons is added to the atomic number, it becomes the mass number (no. of protons + no. of neutrons). The mass number is usually designated as A and is not written in the modern periodic table of elements.

Another property is the atomic mass, which is written below the element name in the periodic table. Due to huge differences between the mass of an electron, and proton and neutron, atomic masses are mostly attributed to the mass of protons and neutrons only (the mass of electrons is almost negligible compared to these two).

 

Quantum Mechanics

If the nucleus lies in the center of the atom, then where do we find the electrons? There are two prevailing models which tried to answer this question. The first one is Neils Bohr’s planetary model, wherein he proposed that electrons revolve around a positive nucleus in a predetermined orbit, just like how the planets in the solar system revolve around the sun.

Later, it was found out that Bohr’s model is lacking in some respects. Then in 1926, Erwin Schrödinger developed his famous equation, which gave birth to the quantum mechanical model. Contrary to Bohr’s planetary model, this model explains that electrons are most probably found in a three-dimensional space around the nucleus, known as the orbital. Schrödinger was able to formulate this using complicated mathematical techniques which require advanced knowledge of calculus to solve. Hence, it will not be discussed here. 

Quantum Numbers

However, it is worth noting that solutions to Schrodinger’s equation are known as quantum numbers, and to completely describe a certain electron in an atom, the four quantum numbers must be specified. If we have a block number, barangay, municipality, and city in our home addresses, electrons, on the other hand, have quantum numbers as their addresses. Below are the four quantum numbers.

1. The Principal Quantum Number (n)

The principal quantum number refers to the main energy levels (or shells) of an orbital. To better understand the principal QN, visualize an imaginary circle around the nucleus of an atom like what is shown below.

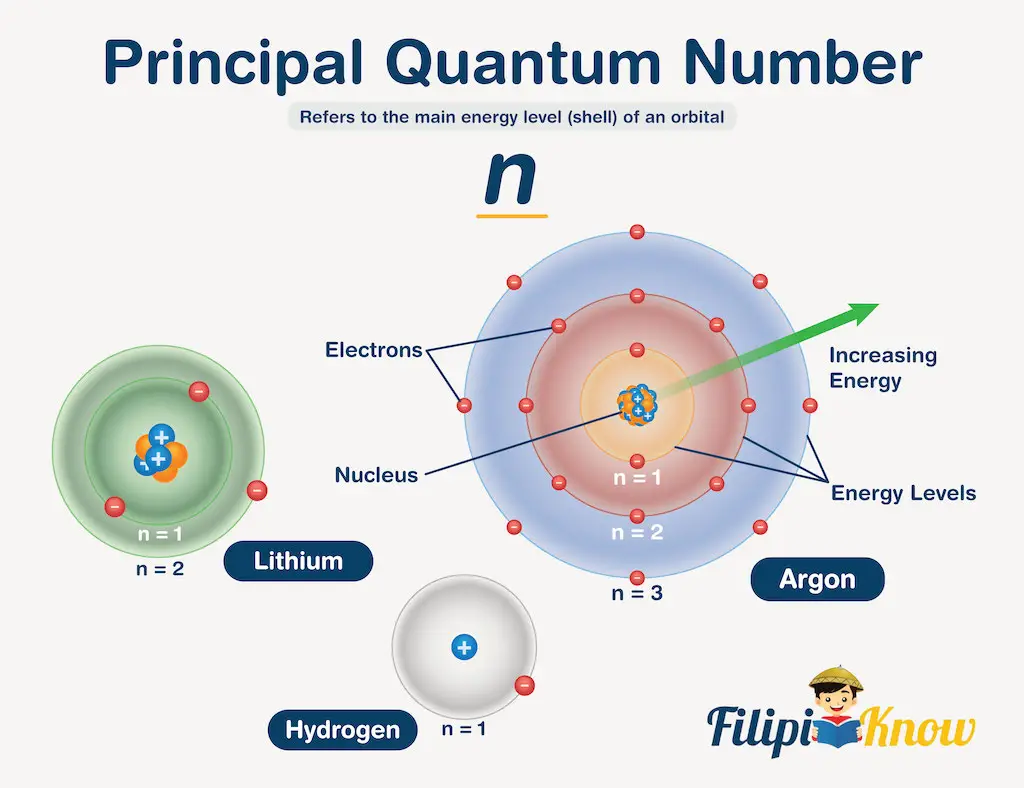
The first circle corresponds to n = 1, and the electrons occupying the n = 1 principal QN can be found anywhere within the space enclosed by the said energy level. This space enclosed by n = 1 is what we call the orbital. The principal quantum number can take values from 1 to ∞, and the higher the value of n, the higher the energy of the orbital, and the farther the electron from the nucleus.

Another thing that the principal QN tells us is the size of the atom. Obviously, the higher the n value, the larger the atom is.

2. Azimuthal Quantum Number (ℓ)

Azimuthal quantum number, also known as the angular momentum quantum number, pertains to the energy sublevels or subshells of the orbital. This quantum number has something to do with the shape of the orbital and can only take integral values between 0 to n-1. Different shapes of orbitals are assigned different letters and ℓ values as shown below.

Letter Designation	Shape	ℓ value
s (sharp)	spherical	0
p (principal)	dumb-bell	1
d (diffuse)	not easily visualized	2
f (fundamental)	not easily visualized	3
G	…	4
…	…	…

This means that ℓ = 0 will always pertain to the s orbital, ℓ = 1 will always pertain to the p orbital, ℓ = 2 will always pertain to the d orbital, and so on.

3. Magnetic Quantum Number (m_ℓ)

Whereas ℓ has something to do with the shape of the orbital, mℓ tells us about the orientation of orbitals in space. The magnetic quantum number takes the values between —ℓ to +ℓ, including zero. Therefore, for an s orbital, the only possible value of m_ℓ is 0, which implies that there is only 1 s orbital. Meanwhile, a p orbital can have an m_ℓ value of —1, 0, and +1, which implies that there are three types of p orbitals, each of which assumes a different orientation in space.

4. Spin Quantum Number (m_s)

Lastly, the spin quantum number pertains to the electron spin, which can only be either clockwise or counterclockwise. As a result, there are only two possible values of m_s, and these are +½ and —½.

We can use these quantum numbers to assign electronic configurations to each electron in a multi-electron system. However, some rules/principles must be followed in assigning electronic configurations.

 

Electron Configuration

The electron configuration is the distribution of electrons among the various orbitals in an atom, molecule, or ion. The electron configuration of hydrogen can be written as follows:

In the orbital diagram, this electron configuration is equivalent to:

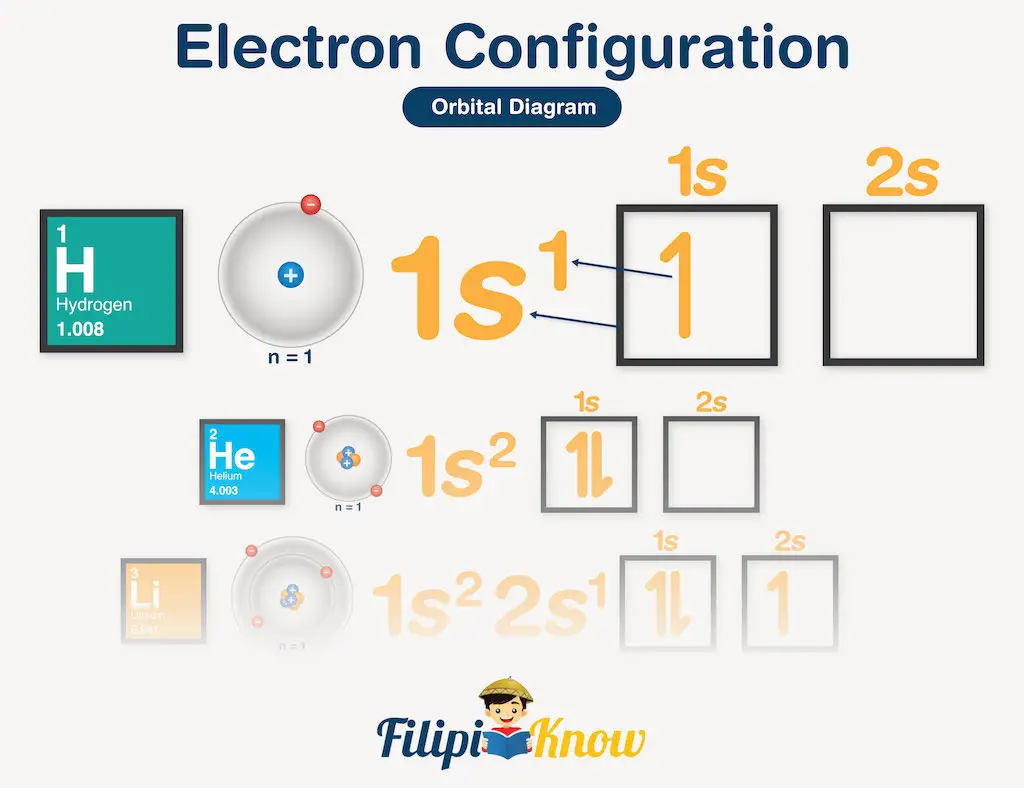
To be able to write electron configuration correctly, it is important that you know three things:

• the number of electrons present in a certain species
• the number of electrons each orbital can occupy
• the correct ordering of the orbitals.

You can easily determine the first one by looking at the periodic table. Recall that the modern periodic table is arranged based on increasing atomic number, and atomic number is equal to the number of protons, so you can also say that for a neutral atom, the atomic number is equal to the number of electrons.

As for the number of electrons each orbital can occupy, just remember that s orbitals can occupy a maximum of 2 electrons, p orbitals can accommodate 6 electrons, d orbitals can take 10 electrons, and f orbitals can have a maximum of 14 electrons. The figure below shows why this is the case.

Recall that m_ℓ pertains to the orientation of orbitals in space and takes the value from -ℓ to +ℓ, including zero. For s orbital, ℓ = 0 so there is only one subshell (each subshell can accommodate a maximum of 2 electrons. For p orbitals, ℓ = 1, so there are 3 subshells (as shown above), and so on.

Lastly, we need to know that we are writing the orbitals in the correct sequence. To do this, you can use the mnemonics presented below:

To have a better understanding of how to use this mnemonic, let us consider the nitrogen atom (Z = 7). Following the flow of arrows in the figure above, the first orbital to use is 1s, followed by 2s, then 2p, 3s, and so on.

We have 7 electrons to accommodate, so the first two can be written as 1s2. The next two electrons occupy the 2s orbital, giving 2s2, and the remaining three will occupy the 2p orbital, giving 2p³. Therefore, the electronic configuration of nitrogen atoms is 1s²2s²2p³ (read as one s two, two s two, two p three). Note that we stopped at 2p orbital because we’re able to accommodate all of nitrogen’s seven electrons by just using up to 2p orbital.

The next question is probably how do we write the orbital diagram of nitrogen? To do this, we need to discuss several principles and rules first.

The Aufbau Principle

Aufbau is a German term that means building up. This principle is named so because it dictates that as a proton is being added one by one to the nucleus to build up the element, electrons are likewise added to the orbital.

For example, a hydrogen atom has 1 proton in its nucleus, and its orbital is occupied by one electron. Adding one proton to hydrogen to form helium will entail the addition of another electron. Such principle gives rise to the question “As more electrons are added to the atom, what orbital will the electron fill up? Do we start with orbitals with the highest energy level, or do we start with the orbitals with the lowest?”

The (n + ℓ) rule answers the dilemma established by the Aufbau principle. According to the (n + ℓ) rule, the added electrons must occupy an orbital with a lower (n + ℓ) value first. In other words, the added electrons should occupy the orbital with lower energy first. This means that as electrons is being added, the 2s orbital (n + ℓ = 2 + 0 = 2) must be filled first before the electrons occupy the 2p (n + ℓ = 2 + 1 = 3) orbital.

In cases wherein two different orbitals have the same (n + ℓ) value, say 3d (n + ℓ = 3 + 2 = 5) and 4p (n + ℓ = 4 + 1 = 5), the orbital with the lower principal QN must be filled first. These principles govern the mnemonics that we used earlier.

When drawing the orbital diagram, we must adhere to Hund’s rule of multiplicity, which states that every orbital in a subshell is singly occupied with one electron before any orbital is doubly occupied, and all electrons in singly occupied orbitals have the same spin.

With what we know so far, we can start to draw the orbital diagram of nitrogen! To do this, it’s best to use the electronic configuration as a guide. Previously, we’ve identified it as:

Nitrogen: 1s²2s²2p³

We know that s orbitals have one subshell, and p orbitals have three subshells. Hence, we can convert this electron configuration to the empty orbital diagram below.

Now, if we are to fill these subshells with electrons, there are two possible scenarios to carry this out, like what is shown below. Both cases use the correct number of electrons in each orbital; however, Case 1 violates Hund’s rule of multiplicity! Case 2 demonstrates what is meant by this rule.

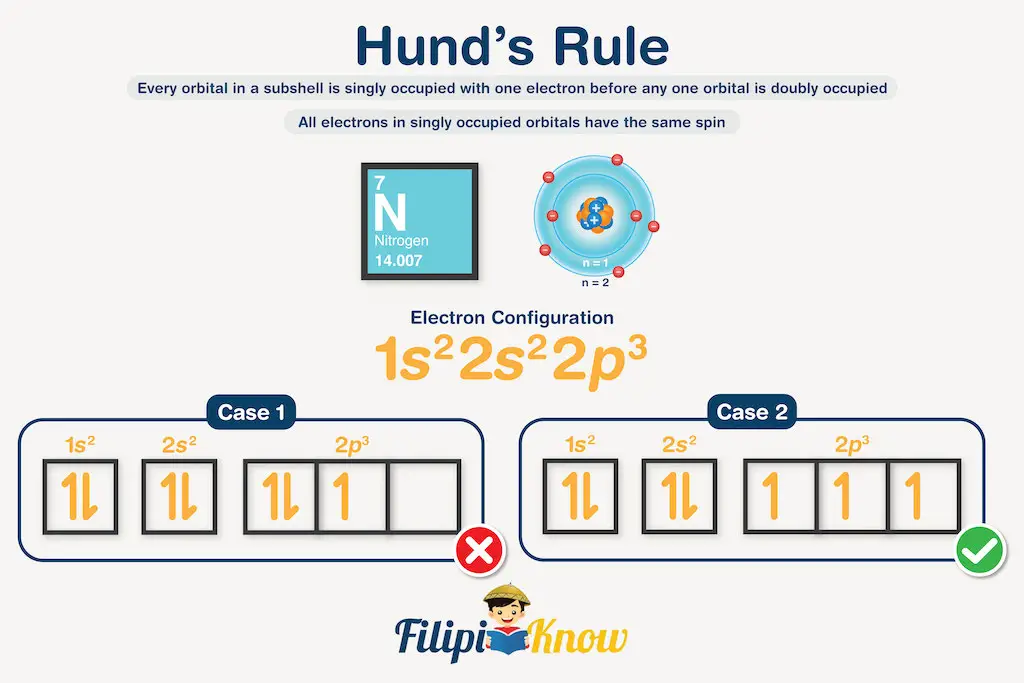
The next question that you might have is: “Why are there arrows pointing upward and downward?” This is a direct consequence of Pauli’s exclusion principle, which states that no two electrons can have the same set of four quantum numbers.

If we consider the two electrons in the 2s orbital, both have the same principal QN (n = 2), azimuthal QN (ℓ = 0 for s orbital), and magnetic QN (m_ℓ = 0). What differentiates the two is the spin QN; one is +½, while the other one is -½. This difference is manifested in orbital diagrams as upward or downward pointing arrows. There is no convention as to which arrow pertains to +½ and -½ spin, although usually, +½ is given to the arrow pointing upward, and -½ to the arrow pointing downward.

Let us work with some examples to better understand these rules and principles in writing electronic configurations.

Sample Problem 1: Write the electronic configuration and orbital diagram for the oxygen atom and assign quantum numbers to the last entering electron.

Solution: The atomic number of oxygen is 8, which means that its electron is also eight. Hence, we need to assign these 8 electrons to 1s, 2s, and 2p orbitals to write the electron configuration, which would be 1s²2s²2p⁴. Using this as a guide for drawing the orbital diagram, we will have:

Due to Hund’s rule of multiplicity, the last entering electron of oxygen is not the one on the rightmost subshell (be very careful with this!), but the one represented by the blue downward arrow in the first subshell of the 2p orbital. With this, we can say that n = 2, ℓ = 1, m_ℓ = -1, and m_s = -½, or simply, (2, 1, -1, -½). Now, let’s try with slightly larger atoms.

Sample Problem 2: Write the electronic configuration and orbital diagram for argon and assign quantum numbers to the last entering electron.

Solution: The atomic number of argon is 18, so we need to distribute 18 electrons to different orbitals. Based on our mnemonics, the correct order of orbitals would be 1s, 2s, 2p, 3s, and 3p. Filling these orbitals with electrons, we will obtain 1s²2s²2p⁶3s²3p⁶. Then, using this electron configuration, we can draw the orbital diagram as follows:

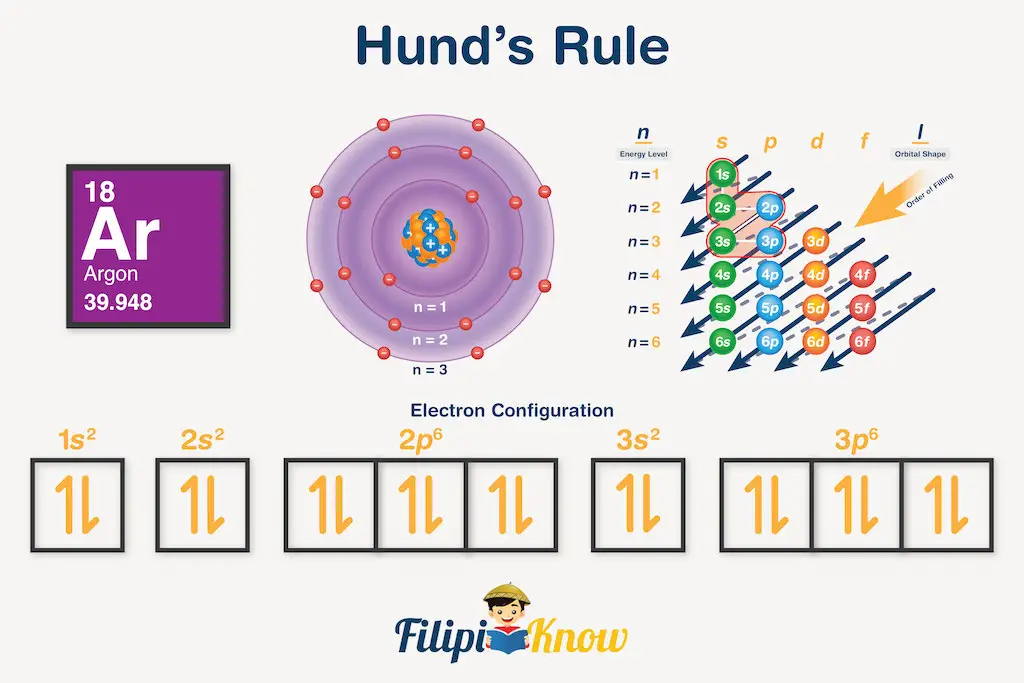
This time, the last downward arrow on the rightmost subshell represents the last entering electron of argon. Since it is located on the third subshell of the 3p orbital, then, n = 3, ℓ = 1, m_ℓ = +1, and m_s = -½, or simply (3, 1, +1, -½).

 

References

Chang, R. (2010). Chemistry (10th ed.). New York City, USA: McGraw-Hill Companies, Inc.

Kotz, J., Treichel, P., & Townsend, J. (2015). Chemistry & chemical reactivity (9th ed.). Stamford, CT: Cengage Learning.

Zumdahl, S., Zumdahl, S., & DeCoste, D. (2010). Chemistry (8th ed.). Belmont, California: Brooks Cole.

 

Next topic: The Periodic Table of Elements and Periodic Trend

Previous topic: Matter

Return to the main article: The Ultimate Chemistry Reviewer

 

Download Article in PDF Format

Test Yourself!

1. Practice Questions [PDF Download]

2. Answer Key [PDF Download]