In studying laws of motion, we found out that there are extraneous variables that act on the object, such as friction.

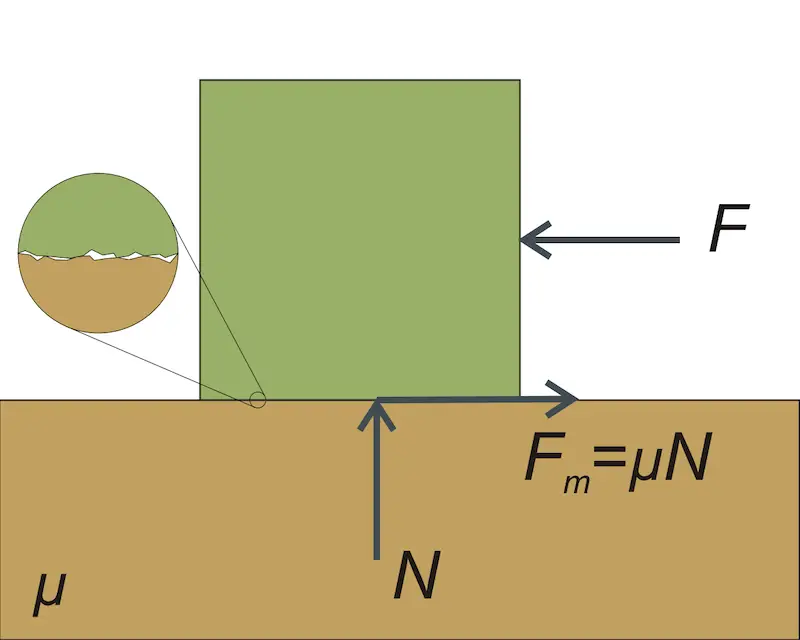
We often neglect it, but it is also an important concept that we have to understand. When an object (say, a block) is in motion along a surface (like ice), the surface exerts a force back upon the object.

The force that is perpendicular to the surface is called the normal force, while the force that is parallel to the surface is the frictional force or, simply, friction.

In this reviewer, you’ll rediscover the principles behind friction and its importance in understanding the laws of motion.

Click below to go to the main reviewers:

Ultimate UPCAT Reviewer

Ultimate NMAT Reviewer

Ultimate LET Reviewer

Table of Contents

• What Is Friction?
• Coefficient of Friction
• Static Friction
• Kinetic Friction
• Rolling Friction
• Sample Problem Involving Friction
• Solution
• Download Article in PDF Format
• Test Yourself!
  • 1. Practice Questions [PDF Download]
  • 2. Answer Key [PDF Download]

 

What Is Friction?

Friction is the force that resists the motion of the object along the surface. Hence, its direction will always oppose the relative motion of the object.

 

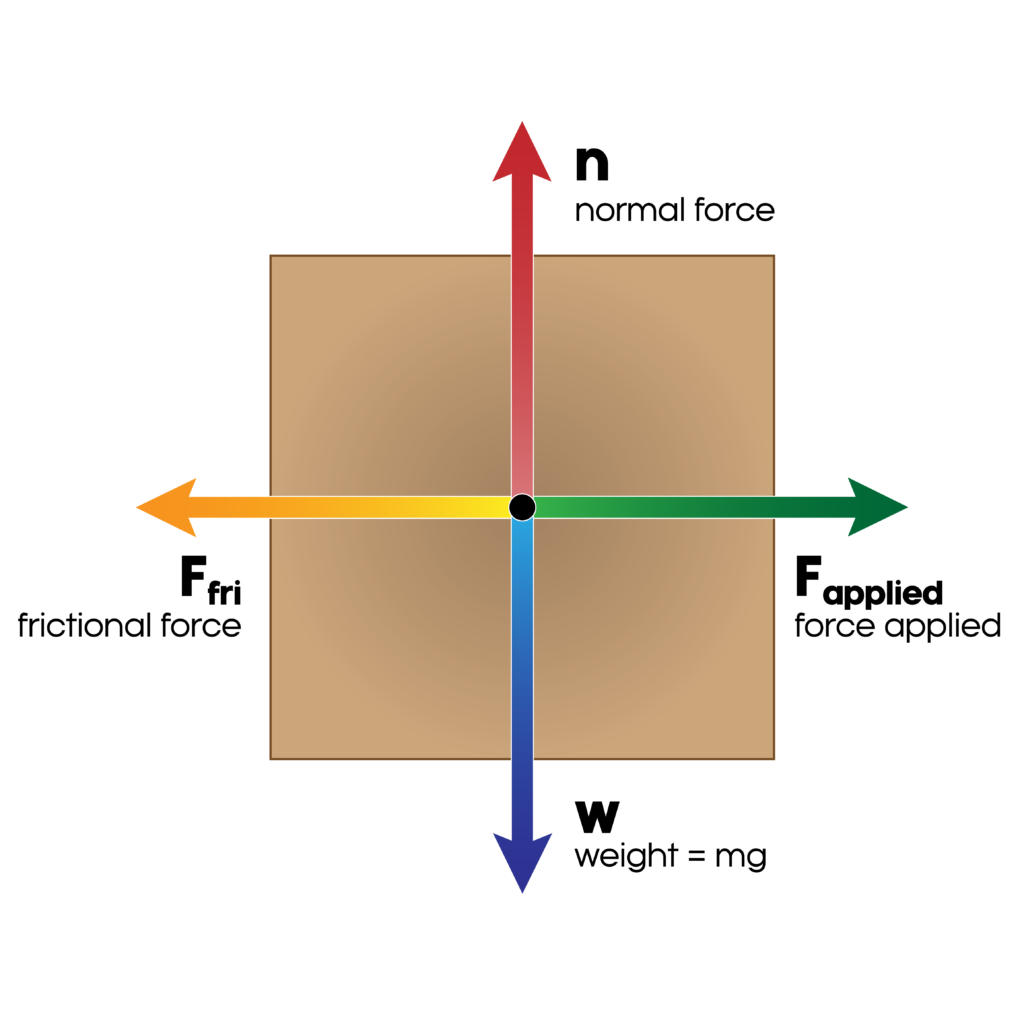
Coefficient of Friction

Every surface has what we call a coefficient of friction which depends on its structure.

If you try sliding a block on some surfaces like sandpaper and ice, what do you notice? Which surface is smoother?

These materials have different compositions, hence, their resistance to motion is also different. The smoother the surface is, the less friction it provides.

However, from the microscopic point of view, even the surface that appears to be smooth has imperfections that provide friction. As the object slides across the surface, there are points where atoms in both the surface and the object interact with one another, causing hindrance to the motion.

The table below shows the coefficient of friction of common surfaces.

Materials	Coefficient of Static Friction, μs	Coefficient of Kinetic Friction, μk
Steel on steel	0.74	0.57
Aluminum on steel	0.61	0.47
Copper on steel	0.53	0.36
Brass on steel	0.51	0.44
Zinc on cast iron	0.85	0.21
Copper on cast iron	1.05	0.29
Glass on glass	0.94	0.40
Copper on glass	0.68	0.53
Teflon on Teflon	0.04	0.04
Teflon on steel	0.04	0.04
Rubber on concrete (dry)	1.0	0.8
Rubber on concrete (wet)	0.30	0.25

 

Static Friction

Try sliding a box across the floor. Were you able to make the box move? It may or may not move because the floor exerts an equal and opposite friction force on the box.

This is called a static frictional force, which is the friction that resists the initiation of the motion. It’s directly proportional to the normal force; hence, the heavier the object is, the greater the normal force and the frictional force. 

Static friction can increase until the magnitude of the applied force is greater than the maximum static frictional force the object exerts, resulting in the acceleration of the box. Its value will be anywhere from zero to the maximum possible value, which can be calculated using

F_max = μ_sn

 

Kinetic Friction

When the applied force exceeds the maximum static friction, the object will begin to accelerate. Once the object is in motion, it now moves against kinetic friction, which refers to the force that opposes relative sliding motion.

Kinetic friction will always be less than static friction since it is harder to get an object going than to keep it moving once you start pushing it. 

The magnitude of the kinetic frictional force acting on a sliding object can be calculated using the formula,

F_k = μ_kn

 

Rolling Friction

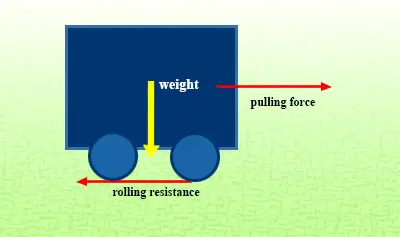
Most of the time, instead of pushing a box to make it slide, you place the box on a cart with wheels to make it move. That is much easier, right? This is because of rolling friction, or the force that resists the motion of a rolling body on a surface. This is equal to

F_r = μ_rn

 

Sample Problem Involving Friction

A 30-kg block of ice rests on the surface. You began pushing it horizontally, and the block started moving when your force exceeds 202 N. You want to keep it moving at a constant 0.31 m/s, reducing your push to 120 N.

Find the coefficients of static and kinetic friction between the block of ice and the surface.

Solution

To understand this further, let us draw a free-body diagram.

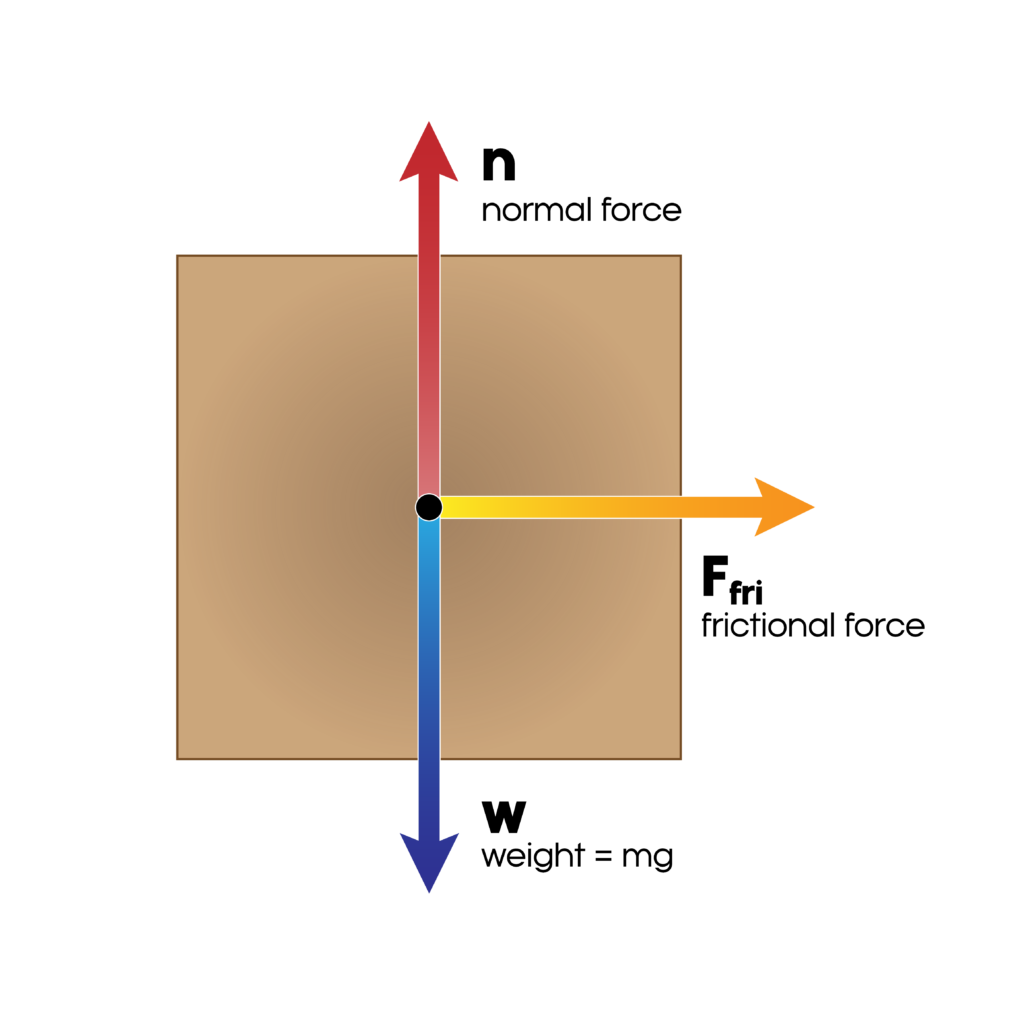
Let us solve for the coefficient of static friction first. We know that this is equal to

F_fr = μ_sN

Using the free-body diagram, we know that

ΣF_y = 0

ΣF_y = n – mg

n = mg

n = (30 kg) (9.8 m/s²)

n = 294 N

ΣF_x = 0

ΣF_x = F – F_fr 

F_fr = F

F_fr = 202 N

Solving for μ_s,

F_fr = μ_sn

μ_s = F_fr/n

μ_s = 202 N/294 N

μ_s = 0.69

For the coefficient of kinetic friction, F_fr = 120 N. Solving for μ_k,

μ_k = F_fr/n

μ_k = 120 N/294 N

μ_k = 0.41

 

Next topic: Work, Energy, and Power

Previous topic: Newton’s Laws of Motions

Return to the main article: The Ultimate Physics Reviewer

 

Download Article in PDF Format

Test Yourself!

1. Practice Questions [PDF Download]

2. Answer Key [PDF Download]