At this point, you’ve probably heard the terms exothermic and endothermic numerous times in the previous modules. In this module, we will discuss not only these two terms but also the thermodynamics of chemical reactions in general.

Click below to go to the main reviewers:

Ultimate UPCAT Reviewer

Ultimate NMAT Reviewer

Ultimate LET Reviewer

Table of Contents

• Energy Changes in Chemical Reactions
• Enthalpy of Reactions
• Standard Enthalpy of Formation and Reaction
  • The Direct Method
  • The Indirect Method
• References
• Download Article in PDF Format
• Test Yourself!
  • 1. Practice Questions [PDF Download]
  • 2. Answer Key [PDF Download]

Energy Changes in Chemical Reactions

Thermochemistry is the study of heat change in a chemical reaction. When we talk about heat, we pertain to the transfer of thermal energy between two different bodies that are at different temperatures. In studying heat changes, there must be a clear boundary between the system or the specific part of the universe where we are interested and the rest of the universe, or the surroundings, where we are not interested.

There are three types of systems, and they are all illustrated in the figure below.

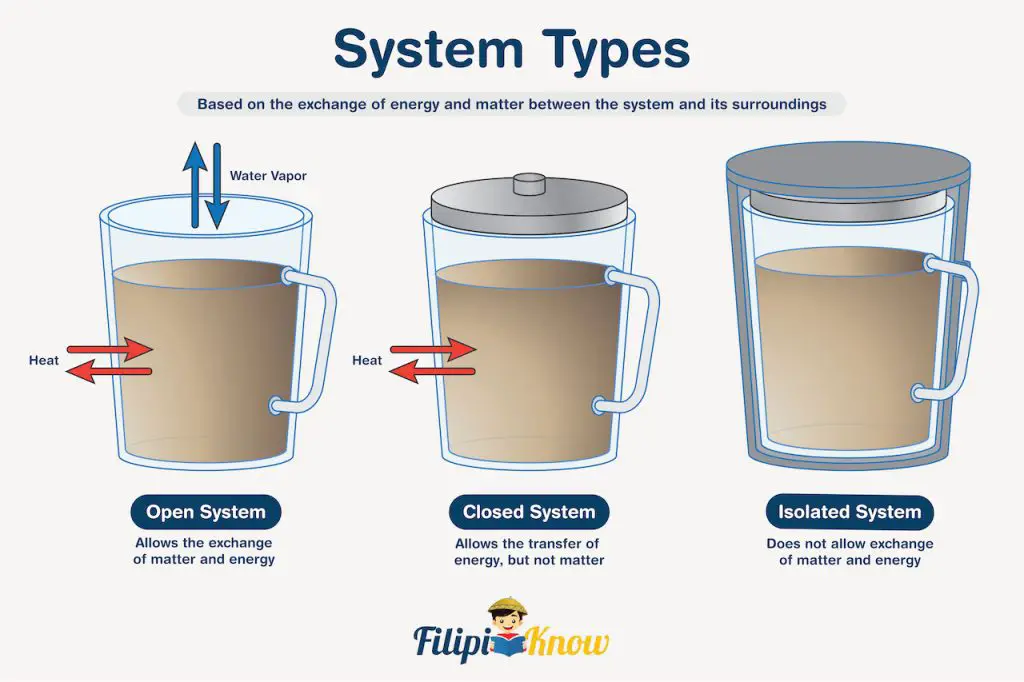
An open system allows the exchange of mass and energy between the system and its surroundings. A cup of hot coffee that was left on top of a table is an example of an open system. The system allows the exchange of energy, hence the coffee cools down through time as it transfers some of its energy to the environment. If left untouched, you can also notice that the volume of coffee decreases because it allows the exchange of matter through the evaporation of hot water.

A closed system allows the transfer of energy but not of matter. If you put a tight lid on the cup of coffee, for example, then it now resembles a closed system. The coffee will still cool down, but its volume will not change since a closed system does not allow the exchange of matter.

Lastly, an isolated system does not permit the exchange of energy and matter. Preparing a coffee in a thermos or an insulated container closely resembles an isolated system. The volume of coffee will not change since there is no exchange of matter, and theoretically, the coffee will remain hot indefinitely because there is no exchange of energy. However, a perfectly isolated system does not exist because there will always be heat flow, so long as there are temperature differences between bodies.

Combustion of fuels is a type of reaction that liberates a considerable amount of heat. The complete combustion of butane is given below as an example.

Because of the law of conservation of energy which states that energy can be transformed from one form to another but can neither be created nor destroyed, the energy generated by the combustion reaction must go somewhere. In this case, the heat generated by the reaction is transferred to the environment. This kind of reaction is called an exothermic reaction.

Other reactions, however, require heat to occur. For instance, the decomposition of HgO occurs only at high temperatures. The reaction is shown below.

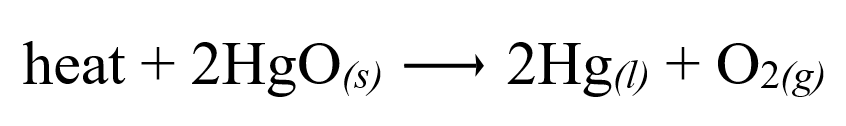
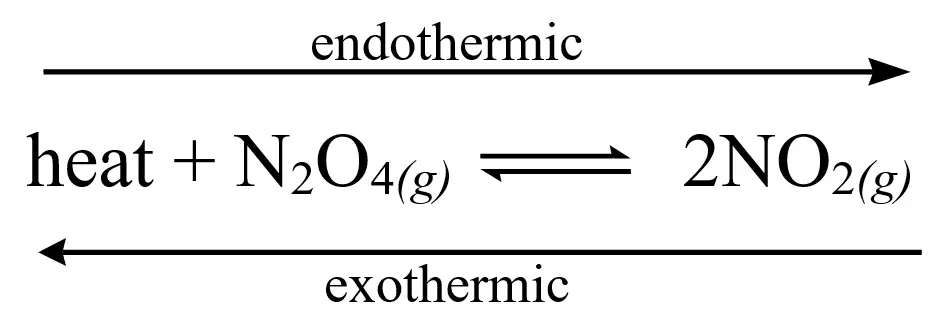
In contrast to the combustion reaction, heat is on the reactant side, meaning the reaction requires heat to proceed. This type of reaction absorbs heat from the environment and hence is termed an endothermic reaction. This can also be applied to reversed/reversible reactions. If the forward reaction is endothermic, then the reverse reaction is exothermic, and vice versa.

Enthalpy of Reactions

Enthalpy (H) is a thermodynamic term used to describe heat changes taking place at constant pressure. For the reactions of the type Reactants → Products, the change in enthalpy (or enthalpy of the reaction, ΔH) can be defined as the difference in enthalpy of the products and reactants. Converting this into a mathematical expression,

ΔH_rxn = H_products – H_reactants

Depending on the type of process, ΔH_rxn can be positive or negative. If the reaction is endothermic, ΔH_rxn > 0. For exothermic reactions, ΔH_rxn < 0. The concept of enthalpy can be applied to two processes: physical or phase change and chemical change.

In phase change, we can consider the conversion of ice to liquid water. From experience, we know that this process absorbs heat from the surroundings, therefore it is an endothermic process, and ΔH_rxn > 0. The equation for this physical change is

H₂O_(s) → H₂O_(l) ΔH = 6.01 kJ/mol

Combustion reactions, on the other hand, are an example of chemical change. As described earlier, combustion reactions are heat-releasing and therefore exothermic with ΔH_rxn < 0. The combustion reaction of butane can be written completely as

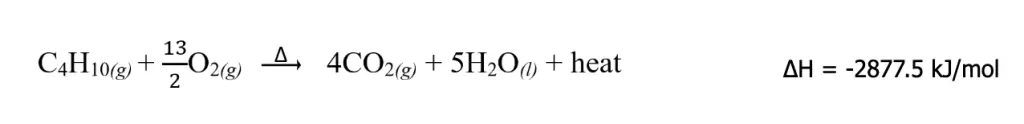
Take note that although the unit of ΔH is expressed on a “per mole” basis, it does not refer to any particular product or reactant. Instead, this means that the corresponding ΔH pertains to the change in enthalpy of the entire reaction as written. Therefore, for the combustion of butane, the following conversion factors can be created.

Equations that show the enthalpy change, as well as the mass relationships of reactants and products, are called thermochemical equations. To avoid errors and discrepancies, several guidelines are used when writing thermochemical equations.

1. It is important to always specify the correct state of each reactant and product. We have learned from the discussion above that phase changes have corresponding ΔH as well. Let us again consider the combustion of butane. If we write H₂O_(g) instead of H₂O_(l), the thermochemical combustion reaction will change accordingly as follows:

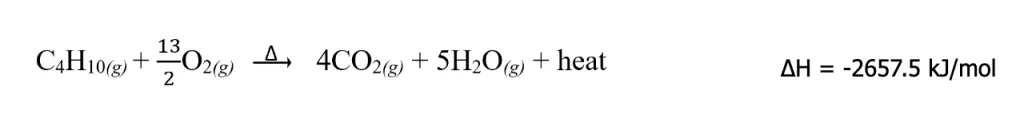
This change is due to the fact that there is an enthalpy associated with the conversion of H₂O_(l) to H₂O_(g).

2. Multiplying the reaction by a certain factor also changes the ΔH by the same factor. To demonstrate this, we know from the discussion above that melting of ice has an associated enthalpy of 6.01 kJ/mol. This value is only true for 1 mole of ice. If the ice becomes 2 moles, the associated enthalpy also doubles.

3. Reversing a reaction or process does not affect the magnitude of enthalpy but reverses its sign. For example, instead of melting ice, let us consider freezing liquid water. By reversing the reaction, we retain the magnitude of ΔH, but its sign will become negative.

This only makes sense because if the original reaction is endothermic (ΔH_rxn > 0), then the opposite process must be exothermic (ΔH_rxn < 0).

Sample Problem:

How much heat must a 45 g of ice absorb for it to melt completely?

Solution:

We know that melting a mole of ice (MM H₂O = 18 g/mol) has an associated enthalpy of 6.01 kJ/mol. From this, we can create the following enthalpy change-mass relationship:

Using stoichiometry, we can easily compute ΔH as follows:

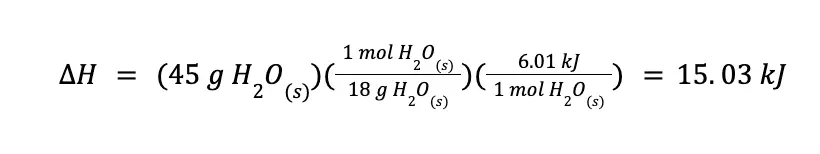
Standard Enthalpy of Formation and Reaction

From the previous discussion, we have seen that the enthalpy of a process or reaction can be obtained by subtracting the total actual enthalpy of the reactants from the total actual enthalpy of the products. The problem is that it is impossible to measure the actual enthalpy of any substance. However, assigning enthalpy values relative to a certain reference is possible.

To achieve this standard value, it is essential that the substance is experiencing some sort of standard condition. For this case, it must be experiencing a 1 atm pressure. The reference point for all enthalpy values is called the standard enthalpy of formation (ΔH°_f). The superscript “°” indicates that the enthalpy indicated is the standard enthalpy.

By convention, the ΔH°_f of an element’s most stable form is zero. Take, for instance, carbon. Due to its valency, carbon exists in many forms, with graphite and diamond as the most popular. Graphite is the most stable form of carbon and hence, assigned the ΔH°_f = 0.

The ΔH°_f of all forms of carbon are based on the ΔH°_f of graphite. For diamonds, it has been determined that ΔH°_f = 1.90 kJ/mole. This value can be interpreted as the heat change that results when 1 mole of a substance (in our case, diamond) forms from its element at 1 atm pressure. It is important that we know this because standard enthalpy of reactions (ΔH°_rxn) can be calculated from the ΔH°_f values.

A hypothetical reaction is shown below.

aA + bB → cC + dD

The standard enthalpy of reaction can be calculated using the formula:

ΔH°_rxn = [cΔH°_f(C) + dΔH°_f(D)] – [aΔH°_f(A) + bΔH°_f(B)]

where a, b, c, and d are the stoichiometric coefficients of a balanced chemical reaction. A, B, C, and D are the identity of the reactants/products, and ΔH°_rxn is the enthalpy of a chemical reaction carried out at 1 atm pressure.

If you look closely, this reaction is simply the enthalpy of the products minus the enthalpy of the reactants, just like what we presented previously. Therefore, the equation can be generalized to

ΔH°_rxn = ∑pΔH°_f(products) – ∑rΔH°_f(reactants)

where p and r are the stoichiometric coefficient of the products and reactants in a balanced chemical reaction, respectively. There are two ways to solve ΔH°_rxn: direct and indirect methods.

The Direct Method

This method of solving for ΔH°_rxn is applicable only for compounds that can be directly synthesized from their elemental compositions. For example, the very famous Haber process (or Haber-Bosch process) is a synthetic process of manufacturing NH₃ from atmospheric nitrogen (N₂) and hydrogen gas (H₂). Nowadays, this reaction is mainly used to synthesize nitrogen-containing fertilizers, although it was originally utilized by the Germans for the production of explosives during World War I. The reaction proceeds as follows:

Both N₂ and H₂ are the most stable forms of nitrogens and hydrogen, respectively. Therefore, based on the convention that we are using, ΔH°_f(N₂) = ΔH°_f(H₂) = 0.

If we desire to determine the ΔH°_f(NH₃), then the calculation is as follows:

ΔH°_rxn = ∑pΔH°_f(products) – ∑rΔH°_f(reactants)

– 91.8 kJ/mol = [2⋅ΔH°_f(NH₃)] – [ΔH°_f(N₂) + 3ΔH°_f(H₂)]

– 91.8 kJ/mol = [2∙ΔH°_f(NH₃)] – [0 + 3∙0]

– 91.8 kJ/mol = [2∙ΔH°_f(NH₃)]

ΔH°_f(NH₃) = -91.8 kJ/mol ÷ 2 = 45.9 kJ/mol

Remember to multiply each ΔH°_f with the corresponding stoichiometric coefficient of each species present in the reaction.

The Indirect Method

Now, if the product/s cannot be formed directly from their elements, we use the indirect method, which is essentially based on Hess’s law of heat summation or simply Hess’s law. This law states that when reactants are converted to products, the change in enthalpy is the same whether the reaction takes place in one step or in a series of steps.

Let us consider the formation of carbon monoxide from graphite (C) and atmospheric oxygen (O₂). The reaction proceeds as follows:

C(graphite) + 1⁄2O_2(g) ⟶ CO_(g)

Unfortunately, burning graphite also produces CO₂ in addition to CO. As a result, the enthalpy change cannot be measured directly, as shown. What we can do instead is to use Hess’s law and the ΔH°_rxn of the two reactions given below, which are known to proceed to completion.

What we can do is reverse one of the reactions such that when we add the two reactions, we will obtain the desired reaction for the formation of CO from graphite and O₂. In this particular example, we can achieve that by reversing the second reaction. However, bear in mind that reversing a reaction also reverses the sign of enthalpy.

To get the overall reaction, we simply add chemical species if they are on the same side or subtract them if they are on the opposite side. In the first reaction, CO₂ is on the product, while it is on the reactant in the 2nd reaction. Therefore we subtract them from one another. Meanwhile, O₂ is also on the opposite side, so we subtract them as well.

To get the ΔH°_rxn of the desired reaction, we simply add the two ΔH°_rxn algebraically, as shown below.

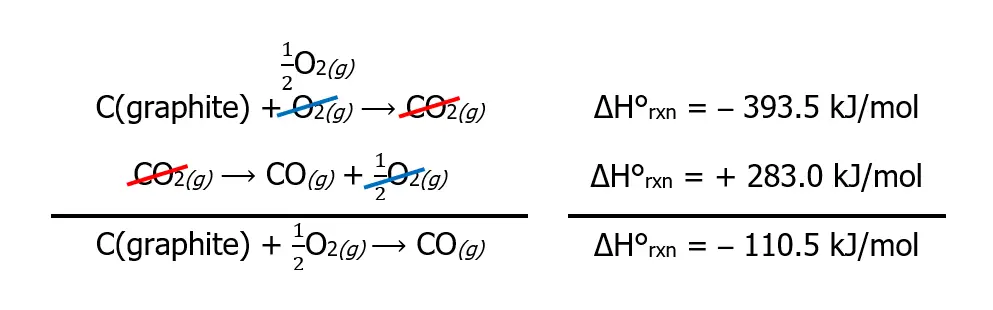
As you can see, we arrived at our desired chemical reaction, and the calculated ΔH°_rxn is -110.5 kJ/mol!

References

Chang, R. (2010). Chemistry (10th ed.). New York City, USA: McGraw-Hill Companies, Inc.

Zumdahl, S., Zumdahl, S., & DeCoste, D. (2010). Chemistry (8th ed.). Belmont, California: Brooks Cole.

Next topic: Nuclear Chemistry

Previous topic: Acids and Bases

Return to the main article: The Ultimate Chemistry Reviewer

Download Article in PDF Format

Test Yourself!

1. Practice Questions [PDF Download]

2. Answer Key [PDF Download]