Have you ever noticed how the trees, structures, and other things seem to be going backward when you look outside a moving bus? Are they really moving backward?

Of course, you know they do not because they are stationary on the ground while the bus that you are riding moves.

How about the people you are with inside the bus?

They appear to be at rest despite moving because you and the other passengers are in the same place (bus). That means there is no relative velocity between you and the passengers.

On the other hand, the trees are stationary when you are moving, which means they move at a relative velocity with respect to the people inside the bus. 

But what is relative velocity?

The relative velocity is the velocity of an object (observer B) in the rest frame of another object (observer A). To understand this concept easier, let us first consider the relative velocity of objects moving along a straight line.

Click below to go to the main reviewers:

Ultimate UPCAT Reviewer

Ultimate NMAT Reviewer

Ultimate LET Reviewer

Table of Contents

• Relative Velocity in One Dimension
• Relative Velocity in Two or Three Dimensions
• Download Article in PDF Format
• Test Yourself!
  • 1. Practice Questions [PDF Download]
  • 2. Answer Key [PDF Download]

Relative Velocity in One Dimension

Suppose an LRT train moves with a velocity of 5 m/s. A passenger walks along the aisle of the train with a velocity of 0.5 m/s. 

What do you think is this passenger’s velocity? 

Sitting on the train, you’ll say that this passenger’s velocity is just 0.5 m/s. However, if you observe outside the train or on another train going in the opposite direction, you will give another answer.

This question is simple, yet there is no concrete answer unless we specify which observer we mean and speak of the velocity relative to a particular observer. This is what we call a frame of reference.

Let’s say you want to find the passenger’s velocity relative to the observer (at rest with respect to the ground) outside the LRT train. To make this simple, we will use A to represent the observer’s frame of reference and B to represent the moving train’s frame of reference.

To solve for velocity, we are going to use the formula 

V_P/A-X = V_P/B-X + V_B/A-X 

Where V_P/A-X is the velocity of P with respect to A; V_P/B-X  is the velocity of P with respect to B, and V_B/A-X is the velocity of B with respect to A.

In this problem, we want to find the passenger’s velocity V_P/A-X with respect to the observer. We know that V_P/B-X  = 0.5 m/s and V_B/A-X = 5 m/s. Performing the calculation, we will get,

V_P/A-X = V_P/B-X + V_B/A-X 

V_P/A-X = 0.5 m/s + 5 m/s

V_P/A-X = 5.5 m/s

In this example, both velocities move in the same direction, which we take as positive. In case the passenger walks to the left relative to the train,  V_P/B-X  = – 0.5 m/s. This makes the passenger’s velocity V_P/A-X with respect to the observer equal to 4.5 m/s.

In case the passenger looks out the window, the observer appears to be moving backward. In this instance, the observer’s velocity relative to the passenger is V_A/P-X which is just the negative of the passenger’s velocity relative to the observer, V_P/A-X. 

In general, if A and B are any two points or frames of reference, V_A/B-X = – V_B/A-X

Sample Problems:

Suppose you are on a bus moving at 35 kph. Your sister sits next to you, and another passenger walks on the aisle at 0.01 kph.

1. What is your relative velocity with respect to the Earth?
2. What is your relative velocity with respect to your sister? 
3. What is the passenger’s relative velocity with respect to the Earth? 
4. What is the passenger’s relative velocity with respect to you and your sister?

Solution:

1. Since you are in a bus moving at 35 kph, your relative velocity with respect to the Earth is 35 kph.
2. Since you are both inside the bus and stationary, your relative velocity with respect to her is 0.
3. Since the passenger is walking on the aisle at 0.01 kph and the bus is moving at 35 kph, the passenger’s relative velocity with respect to the Earth is 35.001 kph.
4. Since you and your sister are sitting on the bus and are stationary, the passenger’s relative velocity with respect to you and your sister is 0.01 kph.

Relative Velocity in Two or Three Dimensions

We often experience instances when one or more objects travel with respect to another observer in a frame that is non-stationary.

A perfect example is a ship crossing a river that flows at some rate or an airplane encountering wind during its motion.

In all these cases, the effect that the medium has on the object must be considered to explain the complete motion of the object.

We measure the relative velocity of the object when doing so, taking into account the particle velocity and the relative velocity.

Sample Problem:

An airplane travels through the air at 280 kph in the North direction. If there is a wind traveling in the East direction at a rate of 45 kph, find the airplane’s velocity relative to the Earth. 

Solution:

In this problem, there are velocities in two dimensions involved: North and East. To solve this problem, we will apply the concept of vectors.

We are given the velocity of the airplane relative to air V_P/A = 280 kph and air velocity relative to the Earth V_A/E = 45 kph. We are looking for the airplane’s velocity relative to the Earth, V_P/E. We know that 

V_P/E = V_P/A  +  V_A/E

Note that these three relative velocities form a right triangle; therefore, we will use vector addition to solve this problem. 

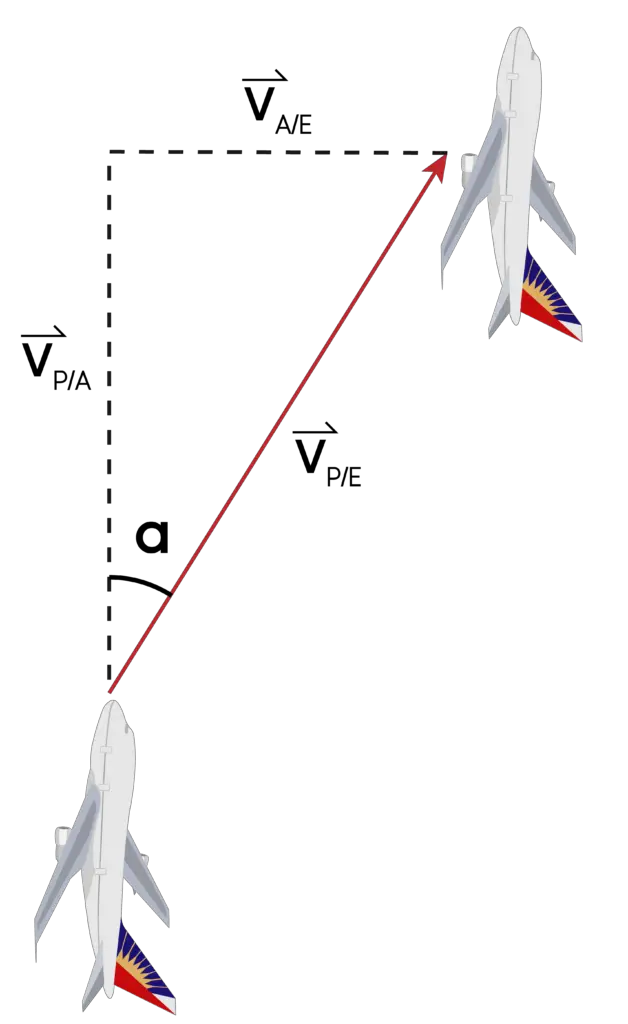
So to answer the question, the airplane’s velocity relative to the Earth is 283.6 kph at 9° East of North. 

Next topic: Laws of Motion

Previous topic: Kinematics

Return to the main article: The Ultimate Physics Reviewer

Download Article in PDF Format

Test Yourself!

1. Practice Questions [PDF Download]

2. Answer Key [PDF Download]