Calculus has two major branches – differential calculus and integral calculus. 

Differential calculus focuses on the concept of derivatives, how to derive them, and their application. We have already covered the basics of differential calculus in the previous reviewer.

This time, let’s jump into the second branch of calculus, which deals with integrals. Just like derivatives, integrals offer a lot of practical applications in various fields. 

But what are integrals? How are they different from derivatives? How can you calculate them?

Let us answer these questions through this reviewer.

Click below to go to the main reviewers:

Ultimate LET Reviewer

Ultimate UPCAT Reviewer

What Are Integrals?

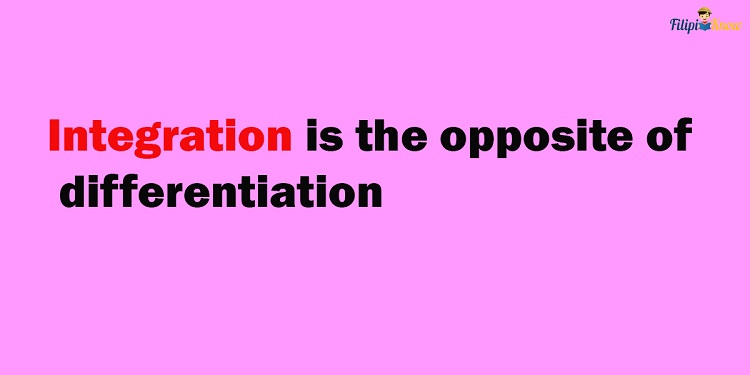
Integrals are the “antiderivative” of a function. This means that the integral is the opposite of the derivative. The process of identifying the integral is known as integration. 

In integration, the given is the function’s derivative, and your goal is to compute the original function. That “original function” is the integral of the given function.

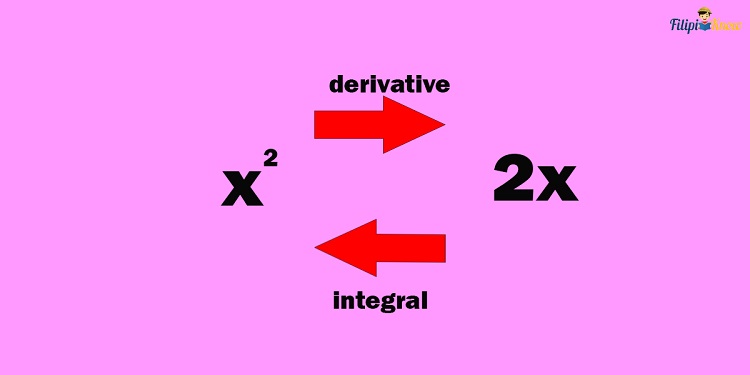
For example, suppose that we want to find the integral of 2x. This means we need to find a function such that its derivative equals 2x. x² is an integral of 2x since the derivative of x² is equal to 2x:

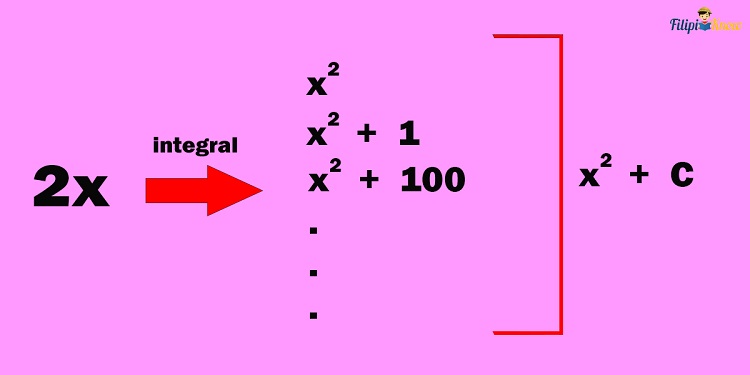
Different functions can be an integral of 2x. For example, x², x² + 1, and x² + 100 are all possible integrals of 2x.

Because a given function takes multiple integrals, we add a letter “C” every time we solve the integral. “C” stands for any arbitrary constant. This is why “C” is called the constant of integration (actually, you can use any letter to represent the constant of integration, but for the sake of convenience, we will use “C” throughout this review to refer to the constant of integration).

Going back to 2x, this function can have multiple integrals. For this reason, we provide a general solution that explains the integral of 2x. Specifically, we express the integral as x² + C, where C is an arbitrary constant. C can take any numerical value such as 1, 1000, ½, π, 0.12, etc.

Since x² + 1, x² + 1000, x² + ½, and x² + π all have a derivative equal to 2x, we just generalize the integral as x² + C.

Integral Notation

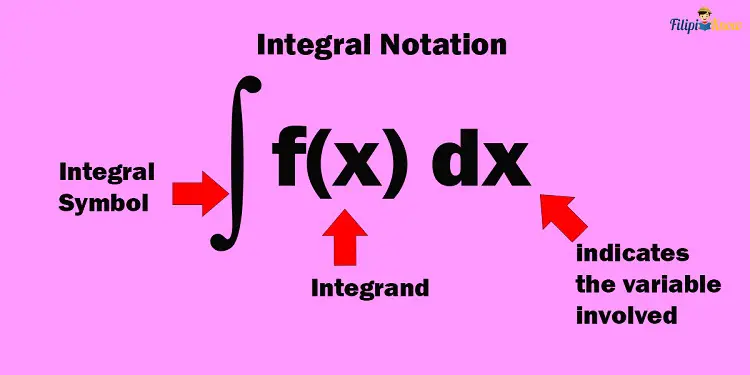
When you see the integral symbol before a given function, it means that we are taking the integral of that function.

The function that we are taking the integral of is called the integrand. Note that there’s always “dx” written after the given function to indicate the variable involved in the integration process (i.e., x).

For example, the notation ∫ 3x² dx means we integrate the function 3x².

We need to identify a specific function whose derivative is equal to 3x². In this case, the integrand is 3x².

Indefinite and Definite Integrals

When taking the integral of a specific function, we must identify whether we are taking its indefinite integral or its definite integral. 

The example above, ∫ 3x² dx, is a case where we are taking an indefinite integral.

In an indefinite integral, there are no upper limits and lower limits involved, and the answer is always a function with a variable and the constant of integration “C.” 

On the other hand, definite integrals are those with upper and lower limits. Let’s take the integral below as an example.

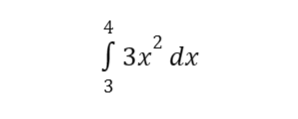
The integral above has small numbers written above and below the integral sign. This is what we refer to as the limits of the integration. It means we are taking a definite integral. In a definite integral, the answer is not a function with a variable x but a whole number.

In this review, we will primarily focus on indefinite integrals. However, we will also provide an overview of definite integrals to prepare you for an actual calculus class.

How To Find the Indefinite Integral of a Function

We apply various integration rules to find the indefinite integral of a function. Note that the indefinite integral of a function is also a function with a variable and contains a constant of integration “C.”

Integration Rules

1. Integral of a Constant

“The indefinite integral of a constant k is kx + C, where x is the variable involved.”

In symbols:

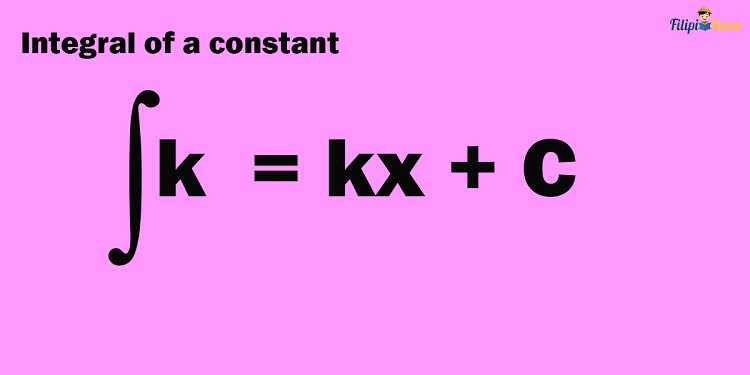
This is the simplest of all the integration rules. It implies that the indefinite integral of a constant (or any numerical value) is just the constant itself times the variable involved in the integration plus the constant of integration C.

Sample Problem 1: Solve for ∫ 2  dx

Solution: To find the integral of 2, we multiply 2 by the variable involved (which is x) and then add C.

Hence, the indefinite integral of 2 is 2x + C. 

Note that the derivative of 2x + C (where C is any numerical value) equals 2. Indeed, 2x + C is the integral of 2. 

Sample Problem 2: Compute for ∫ 9  dx

Solution: Using the integral of a constant rule, we multiply 9 by x and then add C. Hence, the answer is 9x + C. 

Sample Problem 3: What is ∫ π dx?

Solution: Note that the irrational number is also a constant (since it is still a numerical value). Hence, to find ∫ π dx, we need to apply the integral of a constant rule.

To solve for the indefinite integral, we multiply π by x and then add C. Hence, we have πx + C as the answer. 

Sample Problem 4: What is ∫ 9 dm?

Solution: Note that the involved variable this time is not x but m instead. Hence, we should multiply 9 by m and then add C. 

The answer is simply 9m + C. 

2. Power Rule for Integrals

The power rule allows us to identify the integral of a given function with a variable that is raised to a real number value. We can follow the formula below to find the integral of xⁿ (where x is variable and n is a real number). 

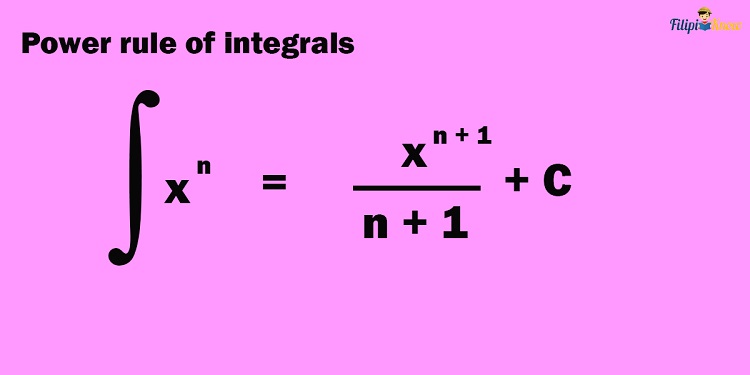
Thus, to find the integral of xⁿ, you must first identify the value of n and then plug it into the formula above.

Sample Problem 1: Determine ∫ x dx

Solution: The given variable here is x with an exponent of 1 (note that x can be written as x¹). This means that we have n = 1. 

Let us plug n = 1 into the formula for the power rule.

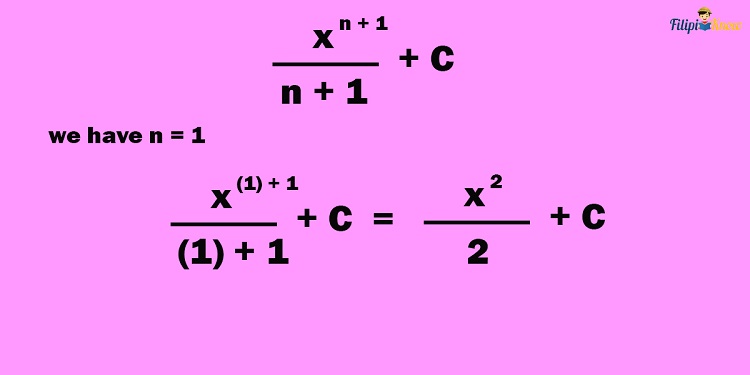
Hence, the indefinite integral of x is:

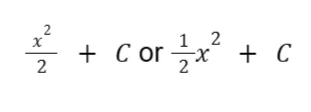
Sample Problem 2: Compute for ∫ x⁵ dx

Solution: The given variable here is x with an exponent of 5. This means that we have n = 5. 

Let us plug n = 5 into the formula for the power rule.

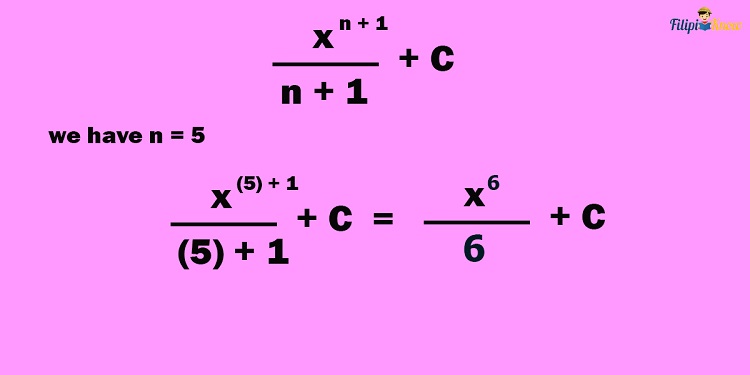
Hence, the indefinite integral of x is:

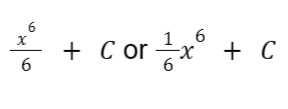
Sample Problem 3: Compute for  ∫ u³ du

Solution: The given variable here is u with an exponent of 3. This means that we have n = 3. 

Let us plug n = 3 into the formula for the power rule.

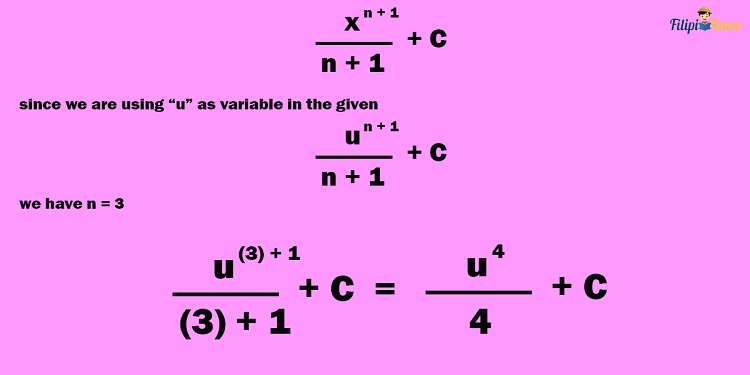
Sample Problem 4: Use the power rule for integration to identify ∫ x^π dx

Solution: The given variable here is x with an exponent of π. This means that we have n = π. 

Let us plug n = π into the formula for the power rule.

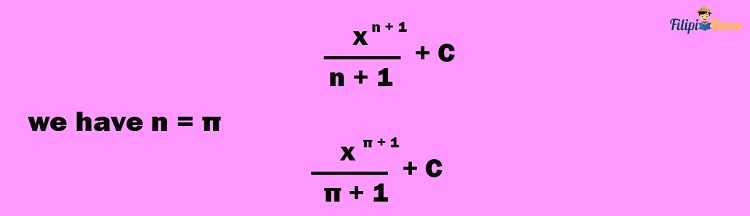
3. Multiplication of a Constant

“The indefinite integral of kxⁿ where k is a constant equals k ∫ xⁿ dx.”

The multiplication of a constant integration rule tells us that the integral of the product of a constant and a function is equal to the product of the constant and the integral of the function.

In symbols: ∫ kxⁿ dx = k ∫ xⁿ dx

Let’s apply this rule to identify the integral of the product of a constant and a function.

Sample Problem 1: What is ∫ 6x² dx?

Solution: The integral of the product of a constant and a function can be expressed as the product of the constant and the integral of the function. This means that we can rewrite ∫ 6x² dx as 6 * x² dx.

The only thing we have to perform now is to compute for ∫ x² dx using the power rule for integrals:

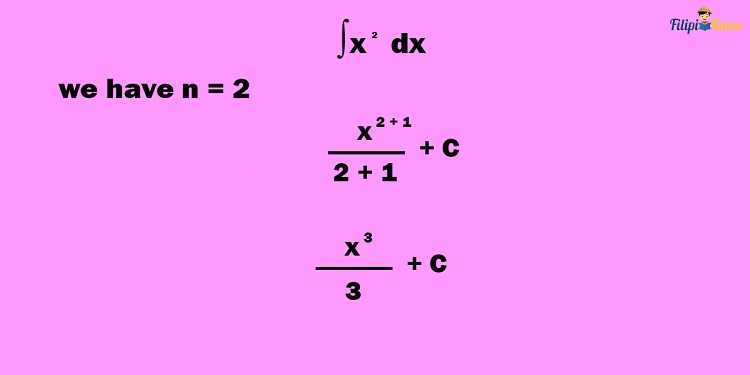
This means that

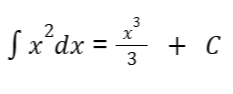
Note that we have already expressed ∫ 6x² dx as ∫ 6 * x² dx. 

Therefore,

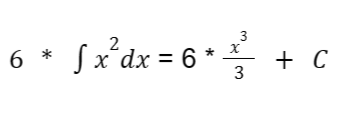
This means that

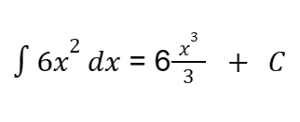
Note that we can write

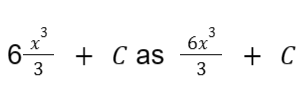
which is also equivalent to

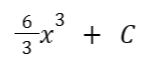
6/3 in lowest terms is ½. Therefore:

The simplified form of

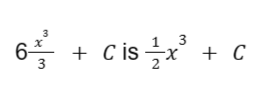
Here’s a quick preview of what we have performed above:

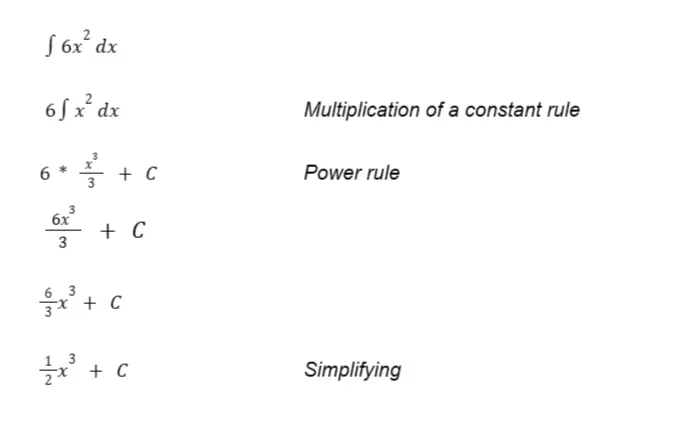
Sample Problem 2: Identify

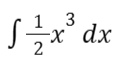
Solution: The integral of the product of a constant and a function can be expressed as the product of the constant and the integral of the function. This means that we can rewrite

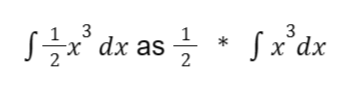
Let us apply the product rule for integrals so that we can identify the value of ∫ x³ dx:

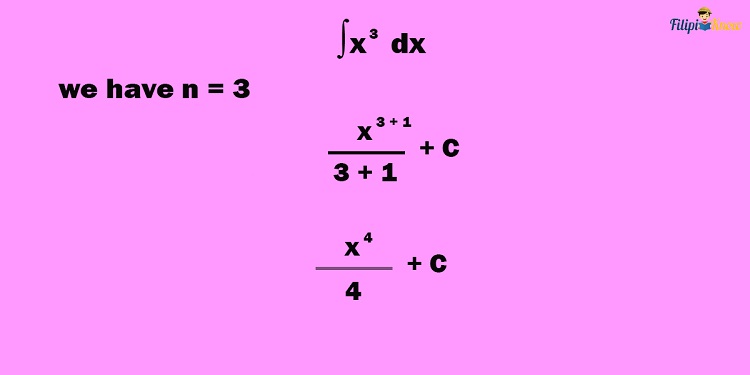
This means that

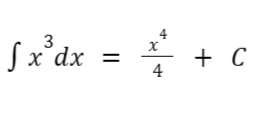
Since we already have ½ * ∫ x³ dx earlier, and we have computed that

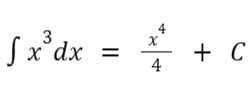
then we have

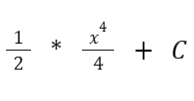
which can be simplified into

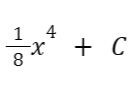
Hence, the answer to this problem is:

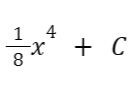
Sample Problem 3: Compute for ∫ 0.10u³ du

Solution: The integral of the product of a constant and a function can be expressed as the product of the constant and the integral of the function. This means that we can rewrite ∫ 0.10u³ du as 0.10 * u³ du.

Let us apply the product rule for integrals so that we can identify the value of ∫ u³ du:

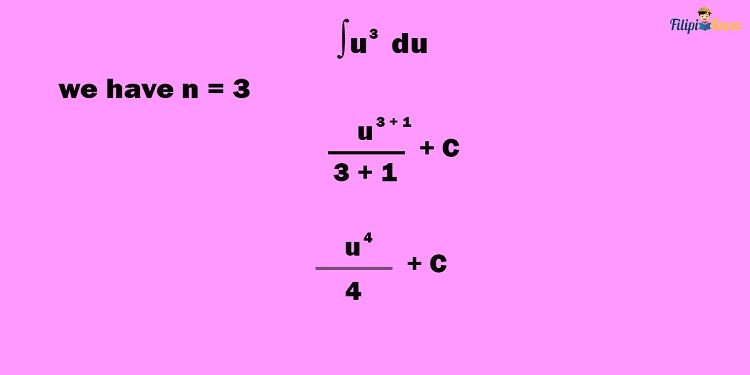
This means that

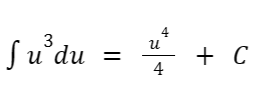
Since we already have 0.10 * ∫ u³ du earlier and we have computed that,

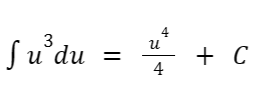
then we have

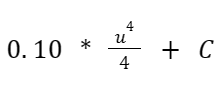
which can be simplified into

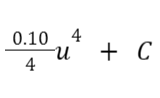
Hence, the answer to this problem is:

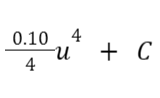
4. Sum Rule of Integrals

“The indefinite integral of the sum of two functions is equal to the sum of the indefinite integral of the functions.”

In symbols, ∫ [f(x)  + g(x)] dx = ∫ f(x) dx + ∫ g(x) dx

The sum rule allows us to find the integral of the sum of the functions by simply rewriting them as the sum of their respective integrals.

Sample Problem 1: Solve ∫ (x² + x )dx using the sum rule.

Solution: In ∫ (x² + x )dx, the integrand is the sum x² + x. This implies that we are taking the integral of the sum of two functions.

By applying the sum rule, we can express ∫ (x² + x )dx as ∫ x² dx + ∫ x dx.

Thus, our next move now is to determine the respective indefinite integrals of x² and x and then add them:

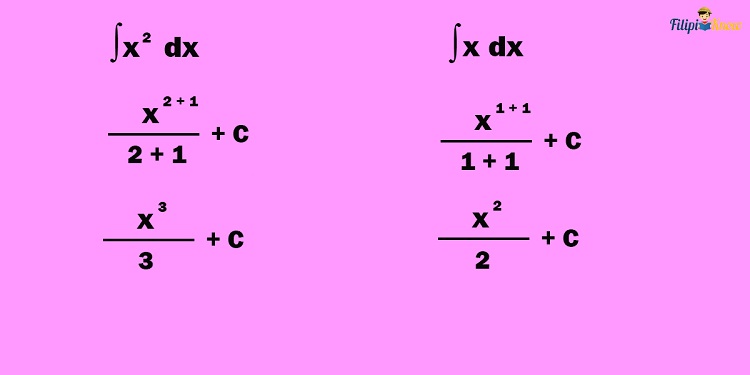
We combine the respective integrals of x² and x and consolidate the two constants of integration as a single constant (since the sum of two constants is also a constant). 

Hence, the answer is

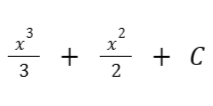
Here’s a quick preview of what we have performed above:

Sample Problem 2: Identify ∫ (2x + x³) dx

Solution: By the sum rule of integrals, we can express ∫ (2x + x³) dx as ∫ 2x dx + ∫ x³ dx. 

Now, let us derive the respective integrals of 2x and x³ :

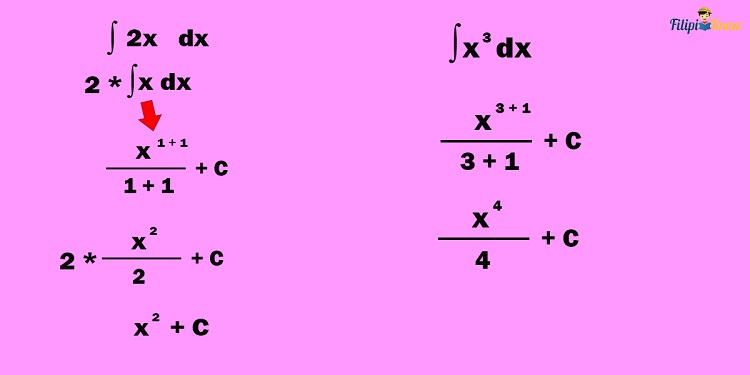
Hence, the answer is

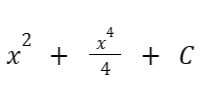
Here’s a quick preview of what we have performed above:

5. Difference Rule of Integrals

This is the counterpart of the sum rule of integrals for subtraction. This rule states that “the indefinite integral of the difference of two functions is equal to the difference of the integrals of the functions.”

In symbols, ∫ [f(x) – g(x)] dx = ∫ f(x) dx – ∫ g(x) dx

Sample Problem 1: Identify ∫ (x² – x⁴) dx

Solution: In the given expression above, the integrand is x² – x⁴, which means we are integrating a difference between two functions. We can apply the difference rule for this case.

According to the difference rule, we can derive the integral of the difference between two functions by simply rewriting them as the difference of the integrals of the functions. Hence, we can rewrite ∫ (x² – x⁴) dx as ∫ x² dx – ∫ x⁴ dx.

Let us now identify the indefinite integrals of x² and x⁴ and subtract them to solve this problem.

Using our solution above, we have determined that the integral is

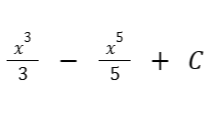
Here’s a quick preview of what we have performed above:

Sample Problem 2: Use the difference rule to identify ∫ (3x³ – ½ x⁴) dx

Solution: Using the difference rule, we can rewrite the given expression ∫ (3x³ – ½ x⁴) dx as ∫ 3x³ dx – ∫ ½ x⁴ dx.

Applying the integration rules we have learned, let us identify the respective integral of 3x³ and ½ x⁴.

Let us start with 3x³. We can express this as 3 * x³.

Using the multiplication of a constant rule, ∫ (3 * x³) is equivalent to 3 * ∫ x³

We can compute the value of ∫ x³ using the power rule for integrals:

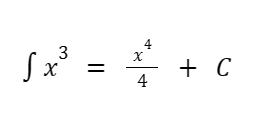
Hence,

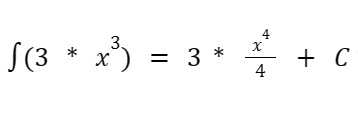
which is equivalent to

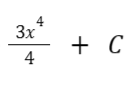
Now, let us find the integral of ½ x⁴.

Using the multiplication of a constant rule, ∫ (½ * x⁴) = ½ ∫ x⁴

We can compute the value of ∫ x⁴ using the power rule for integrals:

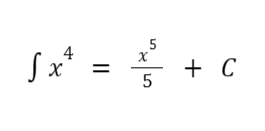
Thus,

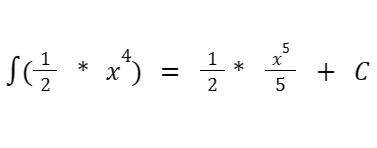
which is equivalent to

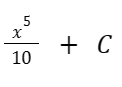
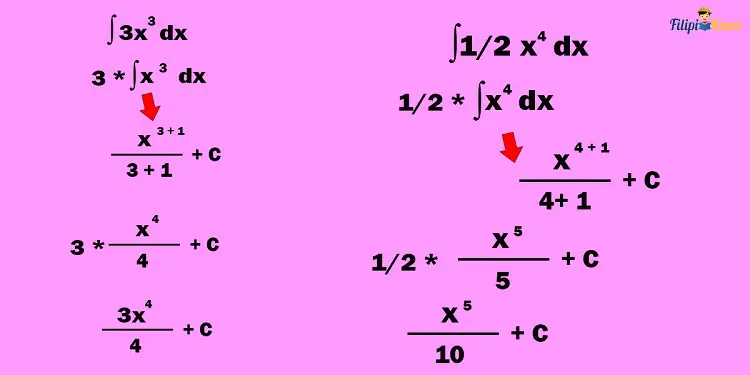
This means that the integral must be

Sample Problem 3: Calculate for ∫ (2x³ + 3x² – x)dx

Solution: Since the given integrand involves addition and subtraction signs, we can apply the sum and difference rules simultaneously.

This implies that we can rewrite the given as ∫ 2x³ dx + ∫ 3x² dx – ∫ x dx

Applying the multiplication by a constant rule, we have as follows:

2 ∫ x³ dx + 3 ∫ x² dx – ∫ x dx

We then apply the power rule to calculate the respective integrals of x³, x², and x:

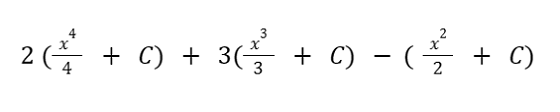
Rewriting the result above, we have the following:

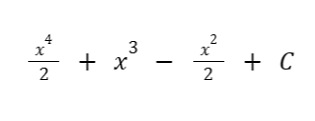
Hence, the integral is:

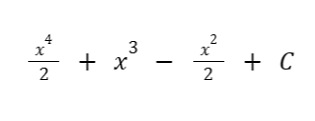
Definite Integrals

In the previous section, we learned how to compute the indefinite integrals of basic functions. Remember that in an indefinite integral, the result is a function with a constant of integration “C.”

On the other hand, a definite integral of a function will give you a specific numerical value. Unlike indefinite integrals, definite integrals don’t have to include the arbitrary constant “C” since we will obtain a specific value in this case. 

By including an upper limit and a lower limit to our integral symbol, we can evaluate the integral and obtain a numerical value.

For instance, the indefinite integral of ∫ 2x dx = x² + C. However, we can obtain a numerical value if we put upper and lower limits to the given expression. 

Suppose that we have

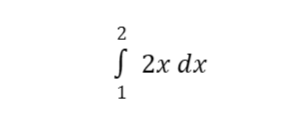
If we solve this, we will obtain 3. Note that we get a numerical value, not a function with an arbitrary constant.

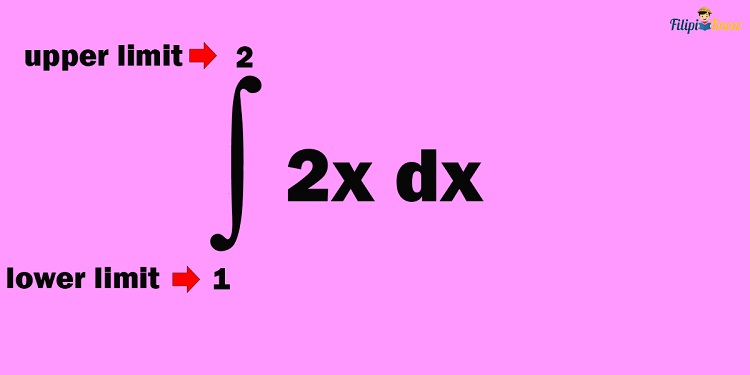
What is this “numerical value” we obtain in a definite integral? This is the area under the curve of the given integrand within the given limits. For instance, in 

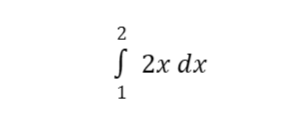
the definite integral of 3 means the area under the function 2x, when graphed in the coordinate plane, is equal to 3 within the range 1 and 2.

We will not delve too much into this analytical concept of integrals because it is already beyond the scope of our reviewer. For this reason, we will be focusing only on the algebra of calculating the definite integral.

How To Compute the Definite Integral

Suppose we want to find the definite integral given a lower limit a and an upper limit b. To find the definite integral:

1. Compute the indefinite integral of the given function.
2. Evaluate the indefinite integral at the lower limit a.
3. Evaluate the indefinite integral at the upper limit b.
4. Subtract the computed value in Step 2 from the calculated value in Step 3.

Sample Problem: Find the definite integral of

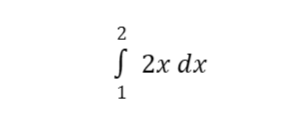
Solution:

Step 1: Compute the indefinite integral of the given function.

By applying the integration rules we learned in the previous sections, we can compute the function’s indefinite integral (2x).

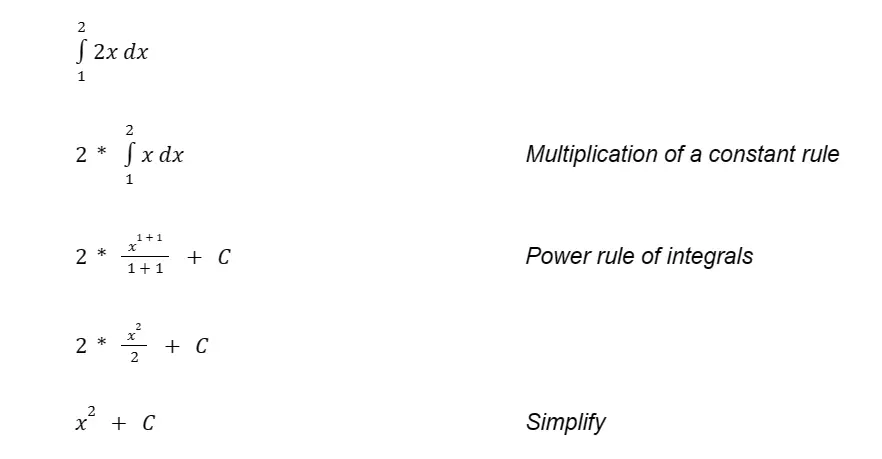
Step 2: Evaluate the indefinite integral at the lower limit a.

The lower limit in the given problem is 1. So, we substitute 1 in the computed indefinite integral in step 1, which is x² + C:

x² + C 

(1)² + C Input the lower limit 1

1 + C

Thus, we have obtained 1 + C.

Step 3: Evaluate the indefinite integral at the upper limit b.

The upper limit in the given problem is 2. So, we substitute 2 in the computed indefinite integral in step 1, which is x² + C:

x² + C 

(2)² + C Input the upper limit 2

4 + C

Thus, we have 4 + C.

Step 4: Subtract the computed value in Step 2 from the calculated value in Step 3.

We obtained 4 + C in step 3 while 1 + C in step 2. Thus, we have:

(4 + C) – (1 + C) = 3

(4 – 1) + (C – C) = 3

Thus, the definite integral is 3. 

Next topic: Propositional Logic

Previous topic: Basic Differentiation

Return to the main article: The Ultimate Basic Math Reviewer

Download Printable Summary/Review Notes

Download Printable Flashcards

Test Yourself!

1. Practice Questions [Free PDF Download]

2. Answer Key [Free PDF Download]

3. Math Mock Exam + Answer Key