The previous chapter taught you how to multiply polynomials. However, you may have realized that multiplying polynomials requires a lot of brute force and is oftentimes cumbersome.

Fortunately, there are some instances when multiplying polynomials becomes easier and more convenient, thanks to different techniques developed by some mathematicians. The result we obtain when multiplying polynomials under special circumstances is called special products.

This reviewer covers the different special products as well as the process of factoring, which is related to special products.

Click below to go to the main reviewers:

Ultimate UPCAT Reviewer

Ultimate NMAT Reviewer

Ultimate PMA Entrance Exam Reviewer

Ultimate PNP Entrance Exam Reviewer

Ultimate LET Reviewer

   

Part I: Special Products

What Are Special Products?

Special products are the result when we multiply polynomials in some special cases, which include:

• Multiplying a binomial by another binomial (FOIL method)
• Squaring a binomial (multiplying a binomial by itself)
• Difference of two squares (multiplying binomials with the same terms but with opposite signs)
• Cubing a binomial (multiplying a binomial by itself thrice)

Don’t worry if you cannot grasp now what each case means. You’ll gradually understand these cases as we go along with this reviewer.

You might also notice that special products seem to be exclusive for binomials. There are also special products with trinomials. However, we will be focusing only on the special cases above since these are the ones that are usually applied in algebra, such as when solving quadratic equations and simplifying rational algebraic expressions.

 

Special Cases Resulting in Special Products

1. Multiplying a Binomial by Another Binomial (FOIL Method)

Let us start with the first special case. This case involves multiplying a binomial by another binomial. 

You have learned from the previous chapter that we can apply the distributive property to multiply these binomials. However, the FOIL method provides us with an easier way to multiply binomials.

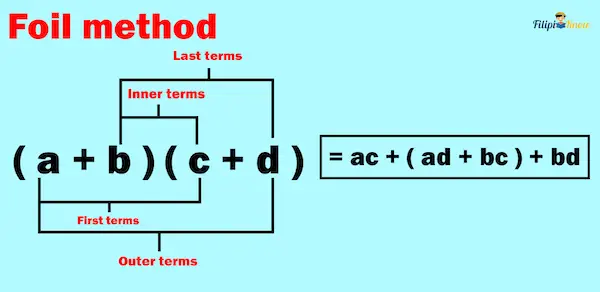
FOIL stands for First Terms, Outer Terms, Inner Terms, and Last Terms. The FOIL method is a technique used to multiply two binomials.

How To Use the FOIL Method in 5 Steps

1. Multiply the first terms of the binomials.
2. Multiply the outer terms of the binomials.
3. Multiply the inner terms of the binomials.
4. Multiply the last terms of the binomials.
5. Combine like terms.

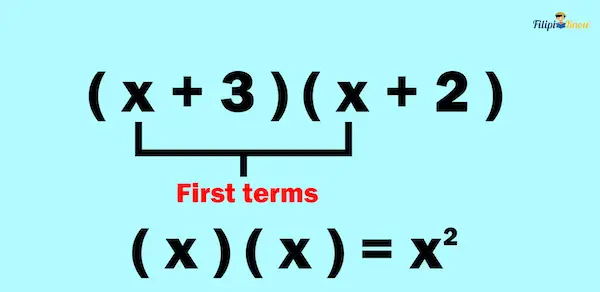
Example 1: Compute for (x + 3)(x + 2).

Solution: Let us use the FOIL method to compute for (x + 3)(x + 2).

Step 1: Multiply the first terms of the binomials. The first terms of the binomials are both x. Hence, x times x is equal to x².

Step 2: Multiply the outer terms of the binomials. The outer terms are the first term of the first binomial and the last term of the second binomial. That is, x times two is equal to 2x.

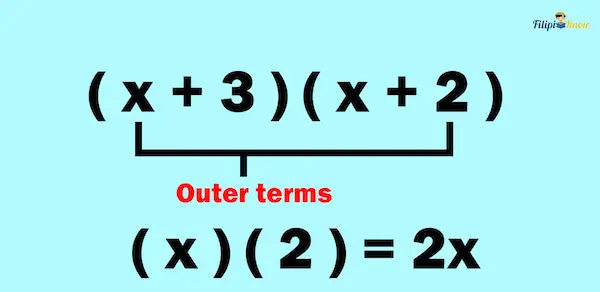
Step 3: Multiply the inner terms of the binomials. The inner terms are the first binomial’s second term and the second binomial’s first term. That is, three times x is equal to 3x.

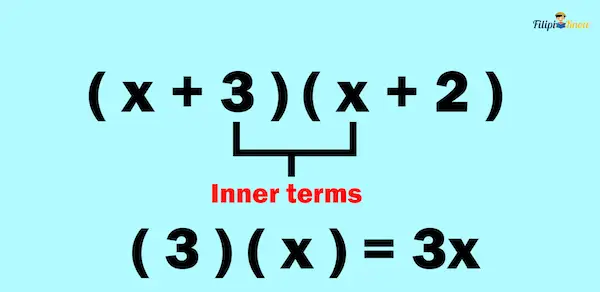
Step 4: Multiply the last terms of the binomials. The last terms of (x + 3) and (x + 2) are 3 and 2, respectively. Thus, 3 x 2 = 6

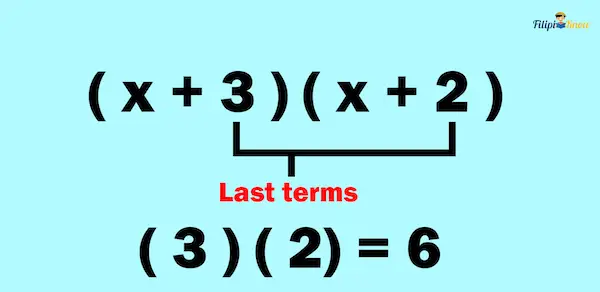
Step 5: Combine like terms. So far, we have obtained x² + 2x + 3x + 6. Note that we can combine 2x and 3x since they are like terms.

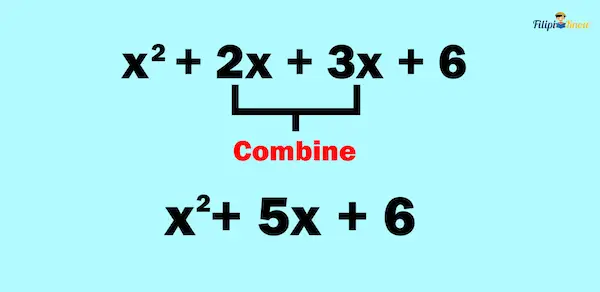
Therefore, using the FOIL method, (x + 3)(x + 2) = x² + 5x + 6

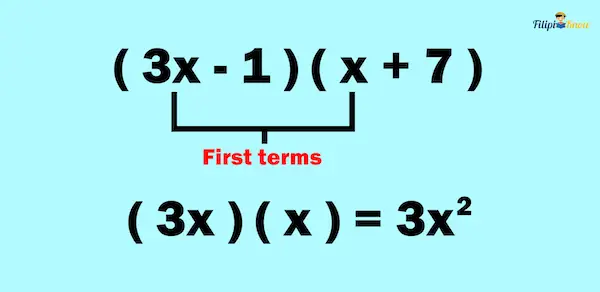
Example 2: Use the FOIL method to multiply (3x – 1) by (x + 7)

Solution:

Step 1: Multiply the first terms of the binomials.

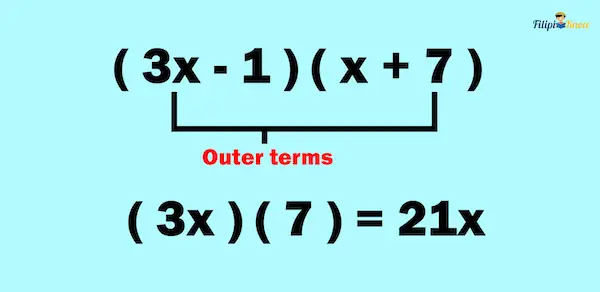
Step 2: Multiply the outer terms of the binomials.

Step 3: Multiply the inner terms of the binomials.

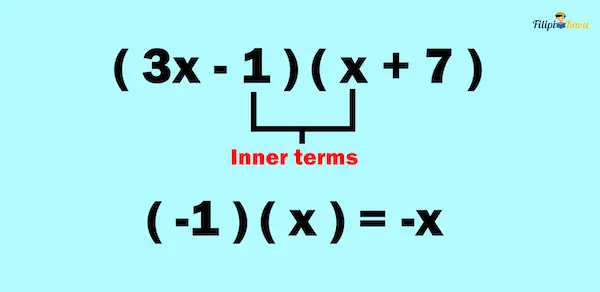
Step 4: Multiply the last terms of the binomials.

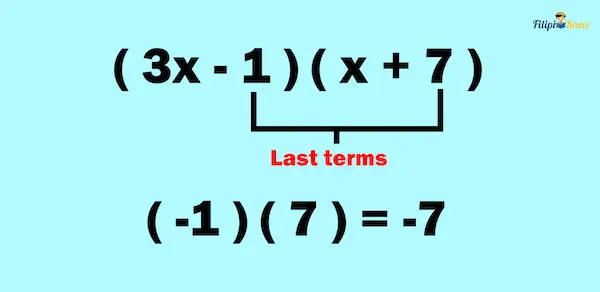
Step 5: Combine like terms

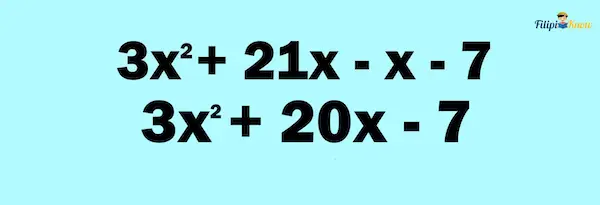
Hence, using the FOIL method, (3x – 1)(x + 7) = 3x² + 20x – 7.

 

2. Squaring a Binomial

In the previous section, you have learned how to multiply a binomial by another binomial. How about if we multiply a binomial by itself?

If we multiply a binomial by itself, we obtain the square of that binomial. For instance, if we multiply (x + 3) by itself, we have this mathematical sentence:

(x + 3)(x + 3) 

We can also express (x + 3)(x + 3) as (x + 3)². (x + 3)² is the square of (x + 3).

Now, what is (x + 3)² or (x + 3)(x + 3) equal to?

You may use the FOIL method to answer this. However, I’ll teach you another technique to determine the square of a binomial. 

Take note that squaring binomial results in a trinomial. If the square of a binomial leads to a trinomial, we say that the square of the binomial is expanded.

How To Square a Binomial in 4 Steps

The square of a binomial (x + y)²  is equal to x² + 2xy + y². 

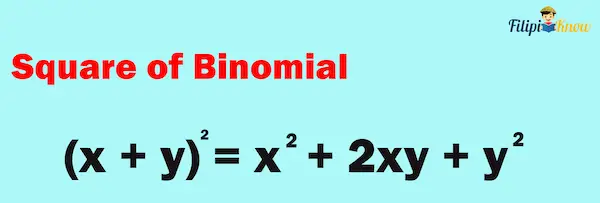
This means that to square a binomial, you should:

1. Square the first term of the binomial.
2. Multiply the first and second term of the binomial then multiply the product by 2.
3. Square the last term of the binomial.
4. Combine the results you have obtained from Step 1 to Step 3.

Example 1: Expand (x + 3)²

Solution:

Step 1: Square the first term of the binomial. The first term of the binomial is x. Squaring x means raising it to the power of 2. Therefore, the square of x is simply x².

Step 2: Multiply the product of the first and second terms of the binomial by 2. The first term is x, while the second term is 3. Multiplying them together, we have 3 times x or 3x. Afterward,  we multiply the result by 2. Therefore, we have 6x.

Step 3: Square the last term of the binomial. The last term of the binomial is 3, and its square is equal to 3² = 9. 

Step 4: Combine the results you have obtained from Step 1 to Step 3. Combining the results we have obtained from the first three steps, we have x² + 6x + 9

Therefore, (x + 3)² = x² + 6x + 9

We have stated earlier that squaring binomial leads to a trinomial. It is important to note that the kind of trinomial you will obtain when you square a binomial is called a perfect square trinomial. x² + 6x + 9 is an example of a perfect square trinomial since it was derived from a square of a binomial, particularly (x + 3)².

Example 2: Expand (p – q)²

Solution:

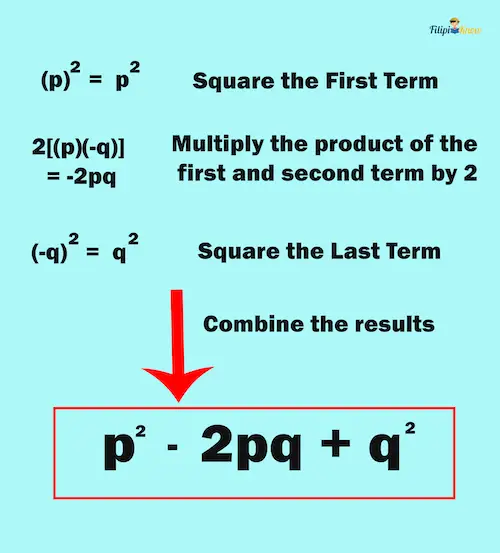
Thus, (p – q)² = p² – 2pq + q²

Example 3: Compute for (2z – 3)(2z – 3)

Solution: We can express (2z – 3)(2z – 3) as (2z – 3)². This means we can apply the steps on squaring a binomial to determine the answer to (2z – 3)(2z – 3).

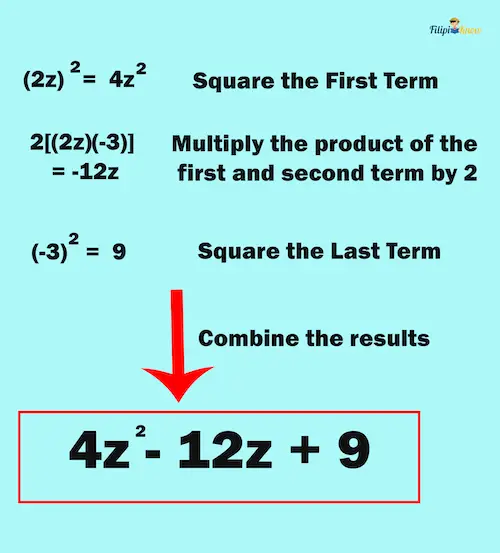
Thus, (2z – 3)(2z – 3) = 4z² – 12z + 9

Example 4: What is the product of (5x – 2)(5x – 2)?

Solution: We can express (5x – 2)(5x – 2) as (5x – 2)². This means we can apply the steps on squaring a binomial to determine the answer to (5x – 2)(5x  – 2).

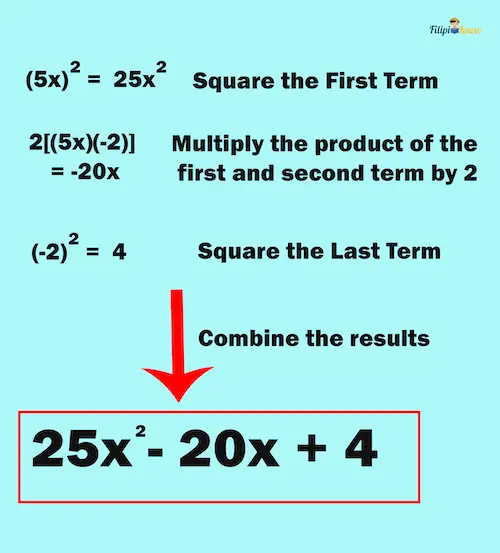
Therefore, (5x – 2)(5x – 2) = 25x² – 20x + 4

As you practice further, you will realize that it is manageable to square a binomial using mental calculation. For instance, let us try to expand (2w – 3)² mentally.

The square of the first term (i.e., 2w) is 4w².

Multiply the first and second terms: 2w times – 3 is -6w. Multiply -6w by 2, and we have -12w.

Then, we square the last term, which is -3: (-3)² = 9

Combining what we have obtained, we have 4w² – 12w + 9.

 

3. Difference between two squares

The difference between two squares is a special product we obtain when we multiply binomials with the same terms but with opposite signs (i.e., one uses a positive/addition sign while the other has a negative/subtraction sign).

For instance, if we multiply (x + y) by (x – y), we will obtain a difference of two squares since (x + y) and (x – y) have the same terms (which are x and y) but opposite signs.

If we multiply binomials with the same terms and one binomial has an addition sign while the other has a subtraction sign, the result is just the square of the first term minus the square of the second term; hence, the term difference of two squares.

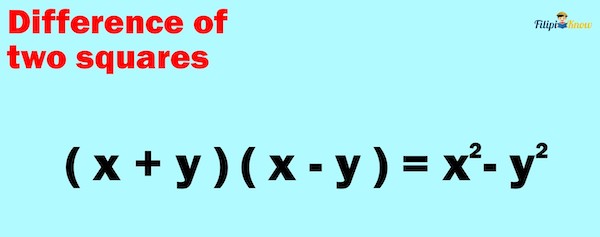
Example 1: Compute for (x + 3)(x – 3).

Solution: Since the binomials have the same terms but with opposite signs, we can conclude that the result will be a difference of two squares.

We square the first term (x) to obtain x2 and square the second term (3) to obtain the answer, so we obtain 9.

Since we have concluded earlier that the result is a difference of two squares, we just put a minus sign between x² and 9.

Therefore, (x + 3)(x – 3) = x² – 9

Example 2: What is the product of (5y – a)(5y + a)?

Solution: Since the binomials have the same terms but with opposite signs, we can conclude that the result will be a difference of two squares.

Squaring the first term: (5y)² = 25y²

Squaring the second term: (a)² = a²

Thus, the answer is 25y² – a²

Example 3: Compute for (a² + b²)(a² – b²)

Solution: Since the binomials have the same terms but with opposite signs, we can conclude that the result will be a difference of two squares.

Squaring the first term: (a²)² = a⁴ (take note that we apply the power rule here)

Squaring the second term: (b²)² = b⁴ 

Therefore, the answer is a⁴ – b⁴

Example 4: Multiply (1 – 3p)(1 + 3p)

Solution:

Squaring the first term: (1)² = 1

Squaring the second term: (-3p)² = 9p²

Therefore, (1 – 3p)(1 + 3p) = 1 – 9p²

Example 5: What is [(x + y) – 2][(x + y) + 2] in expanded form?

Solution: Although three terms seem to be involved in each expression, we can consider (x + y) as a single term since it is grouped using a parenthesis. Thus, we have two terms for each expression and can consider them binomials.

Since the binomials have the same terms but with opposite signs, we can conclude that the result will be a difference of two squares.

Squaring the first term: (x + y)² 

Squaring the second term: (2)² = 4

Thus, we have (x + y)² – 4

However, note that (x + y)² can be expanded further since it is a square of a binomial.

Applying the steps on squaring a binomial. We have: (x + y)² = x² + 2xy + y²

Thus, (x + y)² – 4 = x² + 2xy + y² – 4

Therefore, the answer is x² + 2xy + y² – 4

 

4. Cubing a binomial

Whereas the square of a binomial is the product obtained when we multiply a binomial by itself, a cube of a binomial is what you get when you multiply the same binomial by itself three times.

The cube of a binomial (x + y) can be expressed as:

(x + y)³ = (x + y)(x + y)(x + y)

How to Cube a Binomial in 5 Steps

To find the cube of a binomial:

1. Cube the first term of the binomial (or raise the first term to the exponent of 3).
2. Multiply the square of the first term by the second term, then multiply the product by 3.
3. Multiply the first term by the square of the second term, then multiply the product by 3.
4. Cube the last term (or raise the last term to the exponent of 3).
5. Combine the results you have obtained from Steps 1 – 4.

You must also need to consider the operation used in the binomial. If it’s addition, all of the terms of the expansion are positive. On the other hand, if the operation is subtraction, the second and the last terms are negative, and the rest are positive.

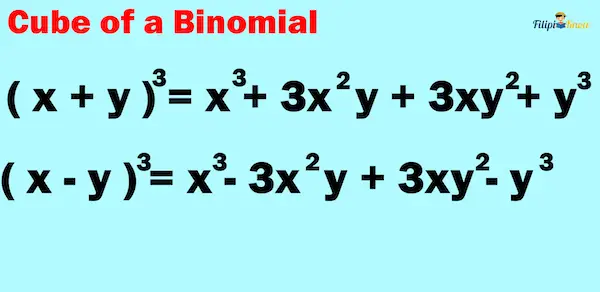
In symbols,

(x + y)³ = x³ + 3x²y  + 3xy² + y³

    (x – y)³ = x³ – 3x²y + 3xy² – y³

Example 1: Expand (a + 1)³

Solution:

Step 1: Cube the first term of the binomial (or raise the first term to the exponent of 3). The first term of the binomial is a. Cube of a is just a³.

Step 2: Multiply the square of the first term by the second term, then multiply the product by 3. The first term of the binomial is a, and its square is a2. We multiply a2 by the second term, which is 1. Hence, a² ✕ 1 = a². Finally, we multiply a² by 3 to obtain 3a².

Step 3: Multiply the first term by the square of the second term, then multiply the product by 3. The first term of the binomial, which is a, must be multiplied by the square of the second term, which is 1 (12 = 1). Thus, we have a ✕ 1 = a. We multiply a by 3 to obtain 3a.

Step 4: Cube the last term (or raise the last term to the exponent of 3). The last term of the binomial is 1, and its cube is just 1³ = 1.

Step 5: Combine the results you have obtained from Steps 1 – 4. Combining what we have obtained from Steps 1 – 4, we have a³ + 3a² + 3a + 1.

Since (a + 1)³ has the addition sign, it means that the terms of the expansions must be all positive.

Therefore, (a + 1)³ = a³ + 3a² + 3a + 1.

Example 2: Expand (2a – b)³

Solution:

Let us apply the steps on how to cube a binomial:

Step 1: Cube the first term of the binomial (or raise the first term to the exponent of 3). The first term is 2a, and its cube is (2a)³ = 8a³.

Step 2: Multiply the square of the first term by the second term, then multiply the product by 3. The first term is 2a, and its square is 4a². We multiply the latter by the second term, which is b. Hence, 4a² ✕ b = 4a²b . Then, we multiply the product by 3: 4a²b ✕ 3 = 12a²b.

Step 3: Multiply the first term by the square of the second term, then multiply the product by 3. The first term of the binomial is 2a. We multiply 2a by the square of the second term (the second term is b, and its square is b²). Thus, 2a ✕ b² = 2ab². Then, we multiply the product by 3 to give the following result: 2ab² ✕ 3 = 6ab²

Step 4: Cube the last term (or raise the last term to the exponent of 3). The last term of the binomial is b, and the cube of b is b³.

Step 5: Combine the results you have obtained from Steps 1 – 4

Since the binomial (2a – b) involves a subtraction sign, the expansion’s second and last terms must be negative, and the remaining terms must be positive.

Therefore, the answer is 8a³ –  12a²b + 6ab² – b³.

 

Part II: Factoring

What Is Factoring?

Factoring is determining the factors of a certain expression or polynomial. We can consider factoring as the reverse process of multiplying polynomials. 

Based on what we’ve learned from the previous chapter in Arithmetic about factors and multiples, factors are the numbers we multiply to obtain the product. Just like numbers, some polynomials also have factors. Through factoring, we will be able to determine what these factors are.

For example, the trinomial x² + 4x + 3 can be factored as (x + 1)(x + 3). Using the FOIL method, you can verify that (x + 1)(x + 3) = x² + 4x + 3

 

Factoring Techniques

We can use different factoring techniques to determine a specific polynomial’s factors. In this section, we will study those techniques one by one:

1. Factoring by the Greatest Common Factor

Do you still remember the Greatest Common Factor (GCF) concept? This section will still apply the same concept to factor polynomials.

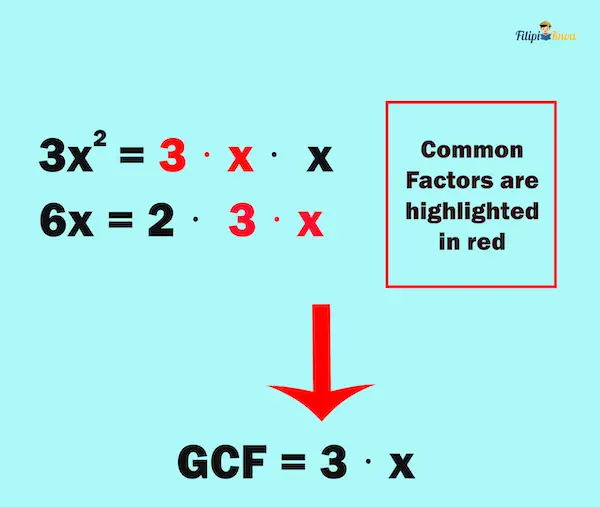
Suppose we have 3x² and 6x. How can we determine the GCF of these expressions?

We need to perform prime factorization on these expressions. To do this, we write the numerical coefficients as a product of its prime factors and the variables with exponents in expanded form.

3x² = 3 ⋅ x ⋅ x

6x = 2 ⋅ 3 ⋅ x

To find the GCF of these expressions, we take the common factors of the expressions and multiply them together. In our list above, note that the common factors are three and x. Thus, the GCF of 3x² and 6x is 3x.

a. How To Factor a Monomial Using the Greatest Common Factor (GCF)

A quicker way to determine the GCF of monomials is by following these steps:

1. Find the GCF of the numerical coefficients.
2. Obtain the common variables and write the smallest exponent among these common variables.
3. Multiply what you have obtained from Steps 1 and 2. The result is the GCF of the monomials.

Example 1: Find the GCF of 35y² and 49y³.

Solution:

Step 1: Find the GCF of the numerical coefficients. The GCF of 35 and 49 is 7.

Step 2: Obtain the common variables and write the smallest exponent among these common variables. The common variable between 35y² and 49y³ is y. We put the smallest exponent among the common variables to y. Notice that the smallest exponent is 2. So we put two as the exponent of y. Thus, we have y².

Step 3: Multiply what you have obtained from Steps 1 and 2. We have obtained seven from Step 1 and y² from Step 2. Thus, the GCF is 7y².

Example 2: What is the GCF of -16x²y³ and 2xy⁴z?

Solution:

Step 1: Find the GCF of the numerical coefficients. The GCF of -16 and 2 is -2.

Step 2: Obtain the common variables and write the smallest exponent among these common variables. The common variables between the given monomials are x and y. The smallest exponent in x is one, while the smallest exponent in y is three. Thus, we have xy³.

Step 3: Multiply what you have obtained from Steps 1 and 2. We have obtained -2 from Step 1 and xy³ from Step 2. Thus, the GCF is -2xy³.

Example 3: What is the GCF of a⁴b³c and ab⁵c²?

Solution:

Step 1: Find the GCF of the numerical coefficients. Both expressions have a numerical coefficient of 1. Thus, the GCF is 1.

Step 2: Obtain the common variables and write the smallest exponent among these common variables. The common variables are a, b, and c. The smallest exponent of a is 1, the smallest exponent of b is 3, and the smallest exponent of c is 1. Thus, we have ab³c.

Step 3: Multiply what you have obtained from Steps 1 and 2. We have obtained one from Step 1 and ab³c from Step 2. Thus, the GCF is ab³c.

Now that you have an idea of how to find the GCF of some monomials. Let us use the same technique to factor polynomials.

b. How To Factor a Polynomial Using the Greatest Common Factor (GCF)

Let us try to factor 15x² + 3 using its GCF. Again, recall that factoring means determining the factors of a certain expression.

The first step is to determine the GCF of the terms of the polynomial. The terms of 15x² + 3 are 15x² and 3. Thus, we need to find the GCF of 15x² and 3.

Using the steps we have learned earlier to find the GCF of monomials (since both 15x² and 3 are monomials), we can obtain the GCF, which is 3.

The next step is to divide each polynomial term by the GCF. 

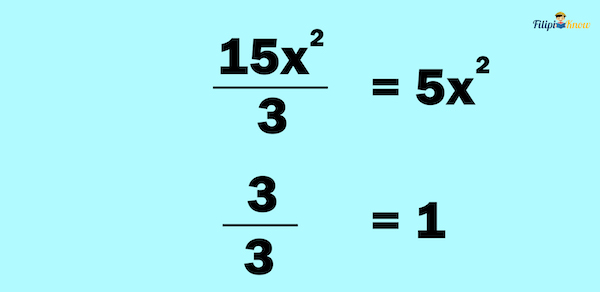
Combining them will give us 5x² + 1. This means that the GCF (which is 3) and 5x² + 1 are the factors of 15x² + 3.

Therefore, if we factor 15x² + 3, we have 3(5x² + 1). Using the distributive property, you can verify that 3(5x² + 1) = 15x² + 3.

The factors we obtained by factoring a polynomial using the GCF are prime factors. They are called as such because we cannot factor them any further. In our previous example, three and 5x² + 1 are prime factors.

Example 1: Factor 14a²b³ – 32a³b

Solution:

The GCF of the terms of the given polynomial is 2a²b.

We divide the terms of the given polynomial by the GCF:

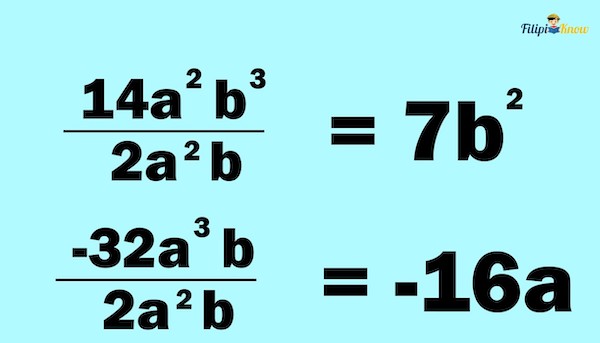
By dividing the terms by the GCF, we can obtain 7b² and -16a. Combining them will give us 7b² – 16a.

This means that the GCF (which is 2a²b) and 7b² – 16a are the factors of 14a²b³ – 32a³b

Therefore, if we factor 14a²b³ – 32a³b, we’ll have 2a²b(7b² – 16a). You can verify using the distributive property that 2a²b(7b² – 16a) = 14a²b³ – 32a³b

Example 2: Factor -21pq²r + 9pqr

Solution:

The GCF of the terms of the given polynomial is -3pqr.

Dividing each term of the given polynomial by -3pqr:

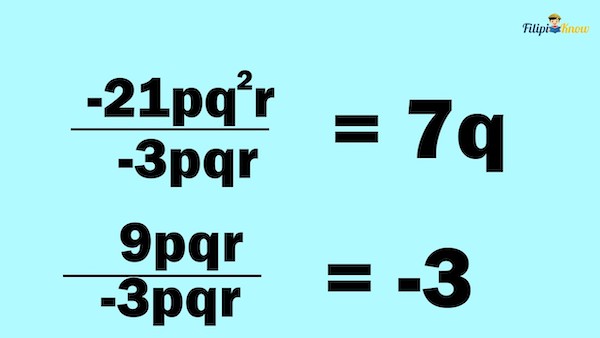
We have obtained 7q and -3. Combining them will give us 7q – 3.

Thus, -21pq²r + 9pqr = –3pqr(7q – 3).

Example 3: Factor ab + ad

Solution:

The GCF of the terms of the given polynomial is a.

If we divide each polynomial term by a, we will obtain b and d, respectively. Combining them will give us b + d.

Therefore, ab + ad = a(b + d). 

Example 4: Factor -12x² + 9x + 3 using the Greatest Common Factor.

Solution:

The GCF of the terms of the given polynomial is -3.

Dividing each term of the polynomial by -3: 

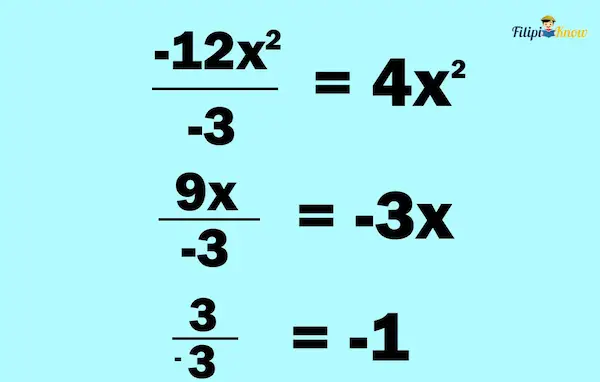
We have obtained 4x², -3x, and -1. Combining them will give us 4x² -3x – 1.

Therefore, -12x² + 9x + 3 = -3(4x² -3x – 1).

Factoring using the GCF is a powerful technique to determine the factors of an expression. However, not every expression is factorable this way. For instance, x² + 6x + 9 is factorable, but its terms’ GCF is 1. We cannot use the steps we have discussed above to factor x² + 6x + 9. The next section will discuss another way to factor an expression.

 

2. Factoring a Quadratic Trinomial

A quadratic trinomial is a trinomial in ax² + bx + c form, where a, b, and c are real numbers, but a is not equal to 0. We usually encounter quadratic trinomials when we perform the FOIL method. For example, if we multiply x + 2 by x + 3:

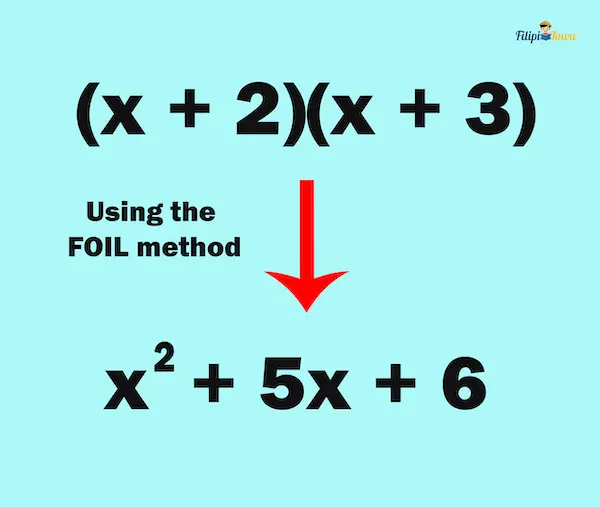
x² + 5x + 6 is an example of a quadratic trinomial since it is in the ax² + bx + c form.

Other examples of a quadratic trinomial are a² + 7a + 10; 4x² + 8x + 1; y² + 4y + 3; and so on.

Example 1: Is 8x² + 2x + x a quadratic trinomial?

Solution: No. Note that we can combine 2x and x since they are like terms. Thus, 8x² + 2x + x is actually 8x² + 3x. 8x² + 3x, although it is quadratic, is not a trinomial.

a. How To Factor a Quadratic Trinomial if a = 1

Let us begin with the simplest type of quadratic trinomials: those whose leading coefficient is one, such as x² + 5x + 6.

A quadratic trinomial can be obtained by multiplying two binomials by the FOIL method. Hence, if we factor a quadratic trinomial, there should be two binomials.

Here are the steps to factor a quadratic trinomial if a = 1:

1. Write the binomials with the first terms as the square root of the leading term of the given quadratic trinomial.
2. Think of the factors of the third term whose sum equals the second term.
3. Write the numbers you have obtained from Step 2 as the second term of the binomials.

Example 1: Let us apply these steps to factor x² + 5x + 6.

Solution:

Step 1: Write the binomials with the first terms as the square root of the leading term of the given quadratic trinomial. We start by writing two binomials with x as the first term.

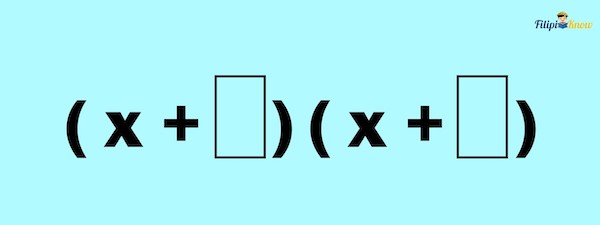
Step 2: Think of the factors of the third term whose sum equals the second term. The third term of x2 + 5x + 6 is 6. Think of the factors of 6 that will give you the second term (5).

Here are the factors of 6 and their sums:

• 1 and 6 (sum: 1 + 6 = 7)
• -6 and -1 (sum: -1 + (-6) = -7)
• 3 and 2 (sum: 3 + 2 = 5)
• -3 and -2 (sum: (-3) + (-2) = -5)

Looking at pairs of factors of 6 above, the sum of 3 and 2 equals the second term, which is 5.

Step 3: Write the numbers you have obtained from Step 2 as the second term of the binomials. In this case, 3 and 2 are the factors of 6 we obtained from Step 2, so these numbers become the second term of the binomials:

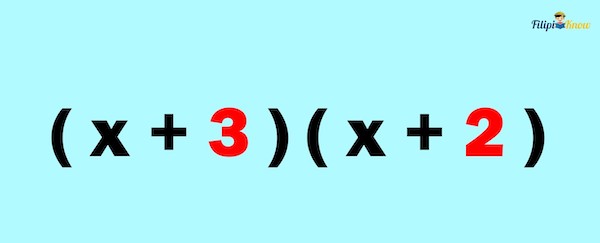
Thus, the factored form of x² + 5x + 6 is (x + 3)(x + 2).

Example 2: Factor x² + 8x + 15.

Solution:

Step 1: Write the binomials with the first terms as the square root of the leading term of the given quadratic trinomial. We start by writing two binomials with x as the first term.

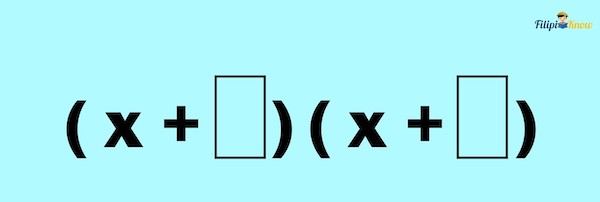
Step 2: Think of the factors of the third term whose sum equals the second term. The third term of x² + 8x + 15 is 15. Think of the factors of 15 that will give you the second term (8).

Here are the factors of 15 and their sums:

• 1 and 15 (sum: 1 + 15 = 16)
• -1 and -15 (sum: -1 + (-15) = -16)
• 3 and 5 (sum: 3 + 5 = 8)
• -3 and -5 (sum: (-3) + (-5) = -8)

Looking at the pairs of factors of 15 above, the sum of 3 and 5 equals the second term, which is 8.

Step 3: Write the numbers you have obtained from Step 2 as the second term of the binomials. In this case, the numbers 3 and 5 each become the second term of the binomials:

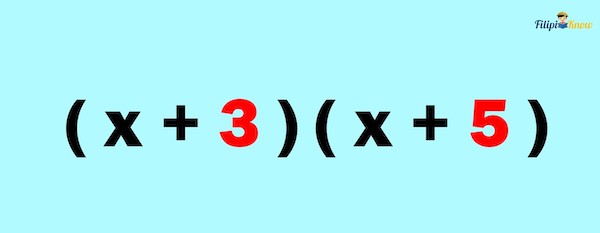
Thus, the factored form of x² + 8x + 15 is (x + 3)(x + 5).

Example 3: Factor x² – 12x + 27.

Solution:

Step 1: Write the binomials with the first terms as the square root of the leading term of the given quadratic trinomial. We start by writing two binomials with x as the first term:

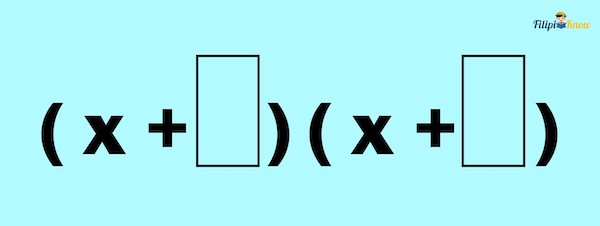
Step 2: Think of the factors of the third term whose sum equals the second term. The third term of x² – 12x + 27 is 27. Think of the factors of 27 that will give you the second term (which is -12).

Here are the factors of 27 and their sums:

• 9 and 3 (sum: 9 + 3 = 12)
• -9 and -3 (sum: -9 + (-3) = -12)
• 27 and 1 (sum: 27 + 1 = 28)
• -27 and -1 (sum: (-27) + (-1) = -28)

Looking at the pairs of factors of 27 above, the sum of -9 and -3 equals the second term of -12.

Step 3: Write the numbers you have obtained from Step 2 as the second term of the binomials. In this case, the numbers -9 and -3 we have obtained from Step 2 become the second term of the binomials:

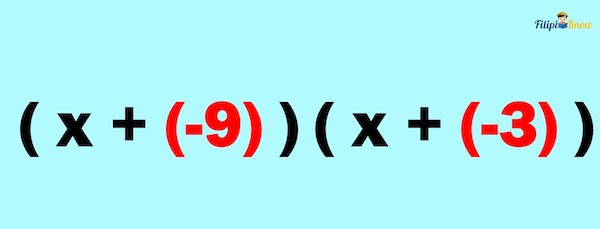
Thus, the factored form of x² – 12x + 27 is (x + (-9))(x + (-3)) or more appropriately, (x – 9)(x – 3).

b. How To Factor a Quadratic Trinomial if a ≠ 1

The previous section discussed factoring quadratic trinomials if a = 1. However, not every quadratic trinomial has a leading coefficient of 1. Most of the quadratic trinomials we will encounter in mathematics have a leading term that is not equal to 1.

So how do we factor quadratic trinomials with a leading coefficient not equal to 1, such as 2x² + 5x + 2; 3x² – 4x + 1; and 6x² – x – 1?

Here are the steps to factor a quadratic trinomial if a ≠ 1:

1. Multiply the coefficients of the first and third terms of the quadratic trinomial. 
2. Think of the factors of the number you have obtained in Step 1, whose sum is equal to the coefficient of the second term.
3. Expand the second term of the trinomial using the factors you have obtained from Step 2. After expanding, the expression should now consist of four terms.
4. Group the trinomial into two groups.
5. Factor out the GCF of each group. Once you have factored out the GCF, expect a common binomial.
6. Factor out the common binomial.

The steps seem to be intimidating, but we will discuss them one by one in our succeeding examples:

Example 1: Factor 3x² – 4x + 1.

Solution:

Step 1: Multiply the coefficients of the first and third terms of the quadratic trinomial. The coefficient of the first term is three, while the coefficient of the third term is one. Multiplying these two numbers to each other will give us the number 3 as the product.

Step 2: Think of the factors of the number you have obtained in Step 1, whose sum is equal to the coefficient of the second term. The number we have obtained from Step 1 is 3. Consider the factors of 3 such that their sum is the coefficient of the second term, which is -4.

Here are the factors of 3 together with their sums:

• 3 and 1 (sum is 4)
• -3 and -1 (sum is -4)

As you can see, the factors of 3 that give -4 as their sum are -3 and -1.

Step 3: Expand the second term of the trinomial using the factors you have obtained from Step 2. After expanding, the expression should now consist of four terms. The second term of the trinomial  3x² – 4x + 1 is -4x. We will expand it by replacing it with the numbers obtained from Step 2. Recall that we have obtained -3 and -1 from Step 2.  Thus, we will replace -4x with -3x and -1x:

3x² – 3x – 1x + 1

Step 4: Group the trinomial into two groups. We group the trinomials using parentheses:

(3x² – 3x) – (1x + 1)

Step 5: Factor out the GCF of each group. Once you have factored out the GCF, expect a common binomial.

We have (3x² – 3x) – (1x + 1). The first group is 3x² – 3x, while the second group is 1x + 1 or x + 1. The GCF of the first group is 3x, while the GCF of the second group is 1. We factor out the GCF of the respective groups:

3x(x – 1) – 1(x – 1)

Note that (x – 1) is the common binomial to 3x(x – 1) – 1(x – 1).

Step 6: Factor out the common binomial. In 3x(x – 1) – 1(x – 1), (x – 1) is the common binomial. We factor it out to complete the factoring process.

(x – 1)(3x – 1)

Therefore, the factored form of 3x² – 4x + 1 = (x – 1)(3x – 1)

Here’s a preview of what we have performed above:

3x² – 4x + 1 

3x² – 3x – x + 1

(3x² – 3x) – (x + 1)

3x(x – 1) – 1(x – 1)

(3x – 1)(x – 1)

Example 2: Factor 2x² + 5x + 2.

Solution:

Step 1: Multiply the coefficients of the first and third terms of the quadratic trinomial. The coefficient of the first term is two, while the coefficient of the third term is two. Their product is 4.

Step 2: Think of the factors of the number you have obtained in Step 1, whose sum is equal to the coefficient of the second term. The number we have obtained from Step 1 is 4. Consider the factors of 4 such that their sum is the coefficient of the second term, which is 5.

Here are the factors of 4 together with their sums:

• 4 and 1 (sum is 5)
• -4 and -1 (sum is -5)
• 2 and 2 (sum is 4)
• -2 and -2 (sum is -4)

As you can see, the factors of 4 that give five as their sum are 4 and 1.

Step 3: Expand the second term of the trinomial using the factors you have obtained from Step 2. After expanding, the expression should now consist of four terms. The second term of the trinomial 2x² + 5x + 2 is 5x. We will expand it by replacing it with the numbers obtained from Step 2. Recall that we have obtained 4 and 1 from Step 2. Thus, we will replace 5 with 4 and 1:

2x² + 4x + x + 2

Step 4: Group the trinomial into two groups. We group the trinomials using parentheses:

(2x² + 4x) + (x + 2)

Step 5: Factor out the GCF of each group. Once you have factored out the GCF, expect a common binomial.

Continuing from the previous step, we have (2x² + 4x) + (x + 2). The first group is 2x² + 4x, while the second is x + 2. The GCF of the first group is 2x while the GCF of the second group is 1. We factor out the GCF of the respective groups:

2x(x + 2) + 1(x + 2)

Note that (x + 2) is the common binomial to 2x(x + 2) + 1(x + 2).

Step 6: Factor out the common binomial. In 2x(x + 2) + (x + 2), (x + 2) is the common binomial. We factor it out to complete the factoring process.

(x + 2)(2x + 1)

Therefore, the factored form of 2x² + 5x + 2 = (x + 2)(2x + 1)

 

3. Factoring a Perfect Square Trinomial

In the previous section, we discussed how to factor quadratic trinomials. However, it is interesting that some special quadratic trinomials can be factored easily without performing the above steps. What are these quadratic trinomials?

A perfect square trinomial is a quadratic trinomial that is derived from squaring a binomial.

Suppose (x + 1)^2, which we know using the techniques in squaring a binomial, equals x² + 2x + 1. Since x² + 2x + 1 was derived by squaring x + 1 (a binomial), then x² + 2x + 1 is a perfect square trinomial.

How can we determine if a quadratic trinomial is a perfect square trinomial?

Simple: Get the square root of the first term and the square root of the third term. Multiply them together, then double it. If the result is equal to the second term of the trinomial, then that quadratic trinomial is a perfect square trinomial.

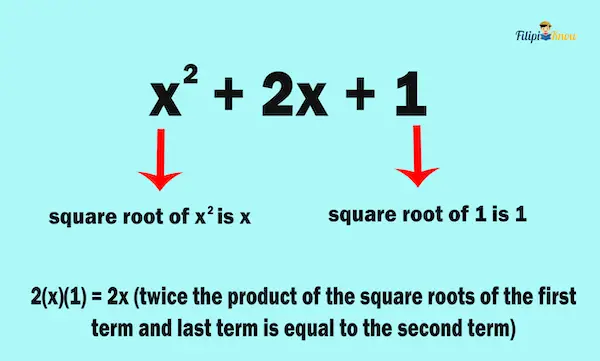
For example, x² + 2x + 1 is a perfect square trinomial. To prove this, we get the square root of the first term (square root of x² is x) and the square root of the third term (square root of 1 is 1). Multiply them (1 multiplied by x is equal to x) and double it (x times 2 is 2x), and the result is equal to the second term (which is 2x). 

Example: Is x² + 6x + 9 a perfect square trinomial?

Solution:

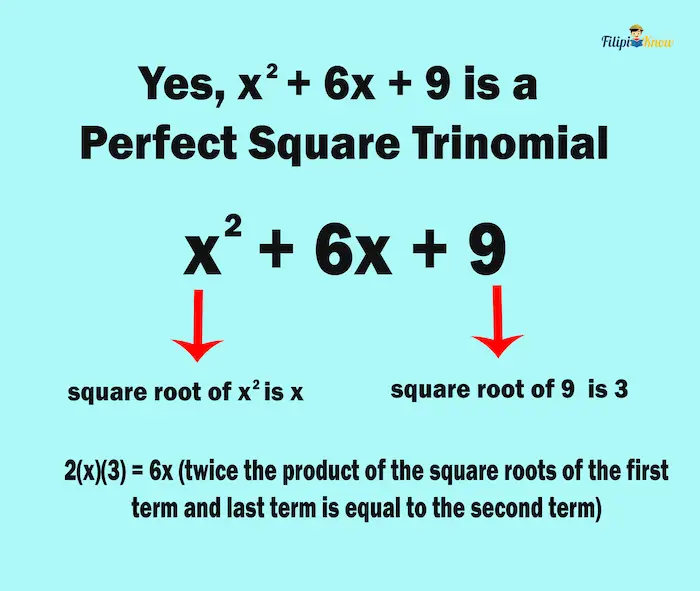
How To Factor a Perfect Square Trinomial in 3 Steps

Once you have confirmed that a quadratic trinomial is a perfect square trinomial, you can factor it using the steps below:

1. Get the square root of the first term. It is the first term of our factors.
2. Get the positive square root of the last term. It is the second term of our factors.
3. The factors will have a subtraction sign if the perfect square trinomial has a subtraction sign. Otherwise, they will be using an addition sign.

Take note that the factors of a perfect square trinomial are two identical binomials.

Example 1: Factor x² + 14x + 49.

Solution:

Step 1: Get the square root of the first term. It is the first term of our factors. The first term is x^2, and its square root is x. Thus, x is the first term of our factors.

Step 2: Get the positive square root of the last term. It is the second term of our factors. The last term is 49, and its square root is 7. Thus, 7 is the last term of our factors.

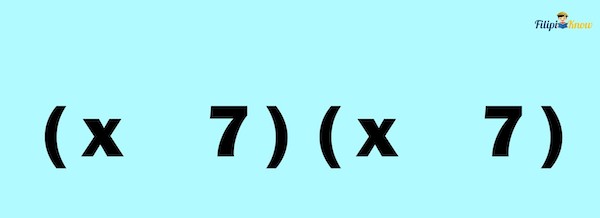
Important Note: When we get the square root of a number, we obtain two values–one is positive, and the other is a negative number. For instance, the square root of 49 is 7 and -7. However, in this case of factoring perfect square trinomials, we will only consider the positive square root of the third term.

Step 3: The factors will have a subtraction sign if the perfect square trinomial has a subtraction sign. Otherwise, they will be using an addition sign.

Since x² + 14x + 49 has no subtraction sign involved, the binomials do not have a subtraction sign but an addition sign.

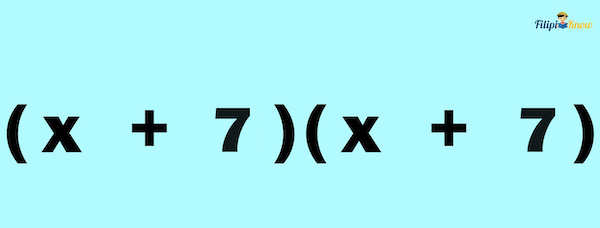
Thus, x² + 14x + 49 = (x + 7)(x + 7)

Example 2: Factor x² – 18x + 81.

Solution:

Step 1: Get the square root of the first term. It is the first term of our factors.

The square root of x² is x.

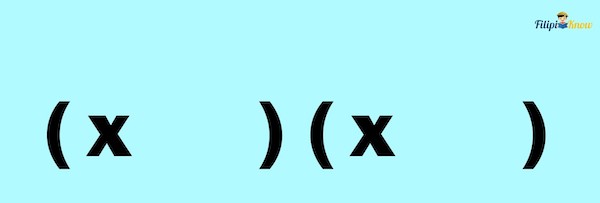
Step 2: Get the positive square root of the last term. It is the second term of our factors.

The square root of 81 is 9.

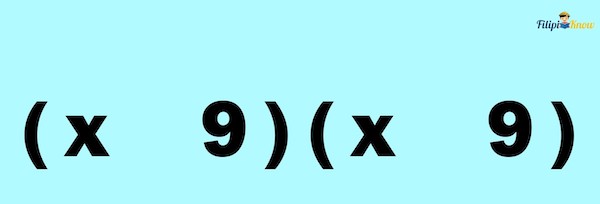
Step 3: If the perfect square trinomial has a subtraction sign, the factors will have a subtraction sign. Otherwise, they will be using an addition sign.

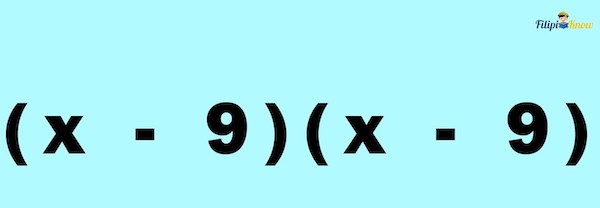
Thus, x² – 18x + 81 = (x – 9)(x – 9)

 

4. Factoring the Difference of Two Squares

A difference of two squares is a binomial in the form a² – b². In the first part of this review, you learned that the difference between two squares is obtained when two binomials with the same terms but with opposite signs are multiplied together.

To factor a difference between two squares:

1. Get the square root of the first term and the square root of the last term.
2. Express the factors as the sum and difference of the quantities you have obtained in Step 1.

Example: Factor a² – 9.

Solution:

Step 1: Get the square root of the first term and the square root of the last term. The square root of the first term is a, while the square root of the second term is 3.

Step 2: Express the factors as the sum and difference of the quantities you have obtained in Step 1. Expressing the quantities we have obtained from Step 1 as sum and difference, we have (a – 3)(a + 3).

Thus, the answer is (a – 3)(a + 3).

 

Next topic: Linear Equations

Previous topic: Polynomials

Return to the main article: The Ultimate Basic Math Reviewer

 

Download Printable Summary/Review Notes

Download Printable Flashcards

Test Yourself!

1. Practice Questions [Free PDF Download]

2. Answer Key [Free PDF Download]

3. Math Mock Exam + Answer Key